一、根据二叉树创建字符串

题目链接：力扣第606题：根据二叉树创建字符串

解析：

这道题主要是关于二叉树的前序遍历，题目要求我们的每一个子树都要用括号括起来，然后要求最终要删除重复的括号，那么我们先来解决最基本的，将括号都括起来再说，然后再讨论如何去除多余括号

我们很容易想到如下的方法可以先将每个都带上括号。就是一个先序遍历，需要注意的就是to_string这个函数也许不是那么的常见，它的作用是将一个整型转换为string类型的。

我们先观察一下测试结果，跟我们预期是一样的。现在主要关心的是除去多余的括号

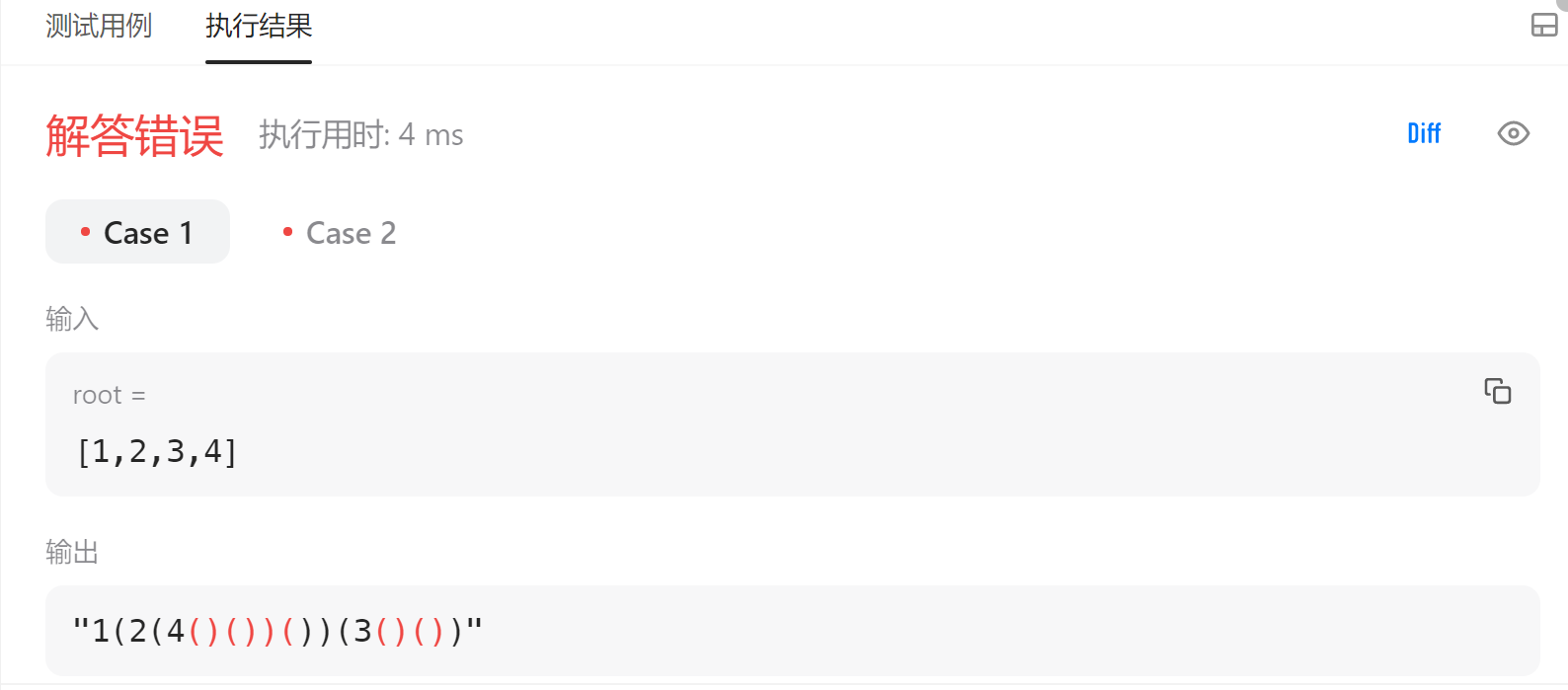
对于除去多余的括号，我们可以先讨论一下，何时加上括号？根据原题的样例，我们不难得知

1. 如果左子树不为空且右子树为空，可以省略右子树的括号，只要输出左子树的括号
2. 如果左子树为空且右子树不为空，不可以省略左子树的括号，左右都要输出
3. 如果两个子树都为空，两个括号都要省略，都不输出左右子树的括号
4. 如果两个都不为空，两个都不省略，左右都要输出

上面的四条规则，根据我们现有的代码，我们可以考虑何时不省略括号的条件即可，只要当不省略的条件满足，就正常加入括号即可。

/*** Definition for a binary tree node.* struct TreeNode {*     int val;*     TreeNode *left;*     TreeNode *right;*     TreeNode() : val(0), left(nullptr), right(nullptr) {}*     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}*     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}* };*/
class Solution {
public:string tree2str(TreeNode* root) {if(root==nullptr){return "";}string str;str+=to_string(root->val);if((root->left&&root->right==nullptr)||(root->left&&root->right)||(root->left==nullptr&&root->right)){str+='(';str+=tree2str(root->left);str+=')';}if((root->left==nullptr&&root->right)||(root->left&&root->right)){str+='(';str+=tree2str(root->right);str+=')';}return str;}
};

上面的代码是可以通过的，不过上面的条件显得过于臃肿了，我们不妨简化一下

/*** Definition for a binary tree node.* struct TreeNode {*     int val;*     TreeNode *left;*     TreeNode *right;*     TreeNode() : val(0), left(nullptr), right(nullptr) {}*     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}*     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}* };*/
class Solution {
public:string tree2str(TreeNode* root) {if(root==nullptr){return "";}string str;str+=to_string(root->val);if((root->left||root->right)){str+='(';str+=tree2str(root->left);str+=')';}if(root->right){str+='(';str+=tree2str(root->right);str+=')';}return str;}
};

二、二叉树的最近公共祖先

力扣链接：力扣第236题：二叉树的最近公共祖先

解析：

对于这道题，如果这道题是一个三叉链的话，就很简单了，因为就相当于退化为了两个链表的相交问题，此时我们直接计算长度，然后使用快慢指针即可。这样就很简单了。不过可惜的是这道题是二叉链的。所以上面的想法是不可以的。

1.解法一：递归

这道题，我们先来画图熟悉一下公共祖先的几种可能性

如上图所示，所有的可能性就两种，一种是如果是4和6的情况下，他们所处的路径上，最近的就是5，此时我们会注意到，对于5这个结点而言，一个在左边，一个在右边。如果是更远一点的公共祖先的话，就不满足这种情况了。要么都在祖先的左边，要么都在祖先的右边。通过这一点，我们就知道了一种祖先的判别方式。还有一种情况就是一个结点是另外一个结点的祖先，如由上图所示。这时候满足的条件就是，如果此时root就等于p或者q的话，那么root就是我们的公共祖先了。

依据上面的分析，我们可以写出如下代码

/*** Definition for a binary tree node.* struct TreeNode {*     int val;*     TreeNode *left;*     TreeNode *right;*     TreeNode(int x) : val(x), left(NULL), right(NULL) {}* };*/
class Solution {
public:bool FindNode(TreeNode* root, TreeNode* obj){if(root==nullptr){return false;}if(root == obj){return true;}return FindNode(root->left,obj)||FindNode(root->right,obj);}TreeNode* lowestCommonAncestor(TreeNode* root, TreeNode* p, TreeNode* q) {if(root == nullptr){return nullptr;}if(root == p || root == q){return root;}bool pInLeft,pInRight,qInLeft,qInRight;pInLeft = FindNode(root->left, p);pInRight = !pInLeft;qInLeft = FindNode(root->left ,q);qInRight = !qInLeft;if(pInLeft&&qInLeft){return lowestCommonAncestor(root->left,p,q);}else if(pInRight&&qInRight){return lowestCommonAncestor(root->right,p,q);}else {return root;}}
};

时间复杂度分析：

这道题的时间复杂度是O(N²)，因为最极端的情况如下所示，即二叉树已经退化为了一个链表，这时候，二叉树高度为N，且由于每次都在一边，这需要N次。在每一次中，又需要判断是不是在左树，这个判断是一个等差数列。所以最终的时间复杂度为O(N²)。不过主要的原因还是在于这是一颗普通的树，假如这棵树是一棵二叉搜索树，那么我们的代价就变小了很多。我们Find的代价直接就没有了，因为根据性质我们就可以知道某个结点是在左子树还是右子树。所以效率变为了O(N)

2.解法二：借助栈来寻找路径

我们一开始想的办法是如果是三叉链的话，那么就可以转化为链表相交的问题，那么如果不是三叉链的话有没有办法能实现类似的思路呢？答案是有的，我们三叉链相比二叉链的优势就是三叉链可以很方便的找到路径。然后根据路径遇到相同的就是最近公共祖先了。

而对于二叉链，我们就只能使用栈来寻找路径了。只要有了路径，那么就可以采用类似的思路了。

所以我们就可以写出如下代码了

/*** Definition for a binary tree node.* struct TreeNode {*     int val;*     TreeNode *left;*     TreeNode *right;*     TreeNode(int x) : val(x), left(NULL), right(NULL) {}* };*/
class Solution {
public:bool FindPath(TreeNode* root, TreeNode* obj, stack<TreeNode*>& st){if(root == nullptr){return false;}st.push(root);        if(obj == root){return true;}if(FindPath(root->left,obj,st)){return true;}if(FindPath(root->right,obj,st)){return true;}st.pop();return false;}TreeNode* lowestCommonAncestor(TreeNode* root, TreeNode* p, TreeNode* q) {stack<TreeNode*> pst;FindPath(root,p,pst);stack<TreeNode*> qst;FindPath(root,q,qst);while(pst.size()>qst.size()){pst.pop();}while(pst.size()<qst.size()){qst.pop();}while(pst.top()!=qst.top()){pst.pop();qst.pop();}return pst.top();}
};

在这段代码中，尤其是寻找路径这段代码，是非常巧妙的，它是利用了返回值的真假来进行截断的。否则我们很难控制路径的结束。

三、二叉搜索树与双向链表

牛客链接：牛客：二叉搜索树转化为双向链表

解析：

这道题我们最容易想到的办法就是暴力法，即直接用一个栈，将每一个结点都放到栈里面。然后再依次将每个结点链接起来即可。不过很可惜，这道题具有空间复杂度要求。显然不想让我们使用任何容器，想让我们就在原地进行修改链接关系。

/*
struct TreeNode {int val;struct TreeNode *left;struct TreeNode *right;TreeNode(int x) :val(x), left(NULL), right(NULL) {}
};*/
class Solution {
public:void InOrder(TreeNode* root, TreeNode*& prev){if(root == nullptr){return;}InOrder(root->left,prev);root->left = prev;if(prev){prev->right = root; }prev = root;InOrder(root->right,prev);}TreeNode* Convert(TreeNode* pRootOfTree) {if(pRootOfTree==nullptr){return nullptr;}TreeNode* ret = pRootOfTree;while(ret->left){ret = ret->left;}TreeNode* prev = nullptr;InOrder(pRootOfTree,prev);return ret;}
};

如上代码所示，既然要是排序的双向链表，那么必然是中序的。在中序的过程中，我们需要改变链接关系，只传一个指针是肯定不够的，虽然我们是无法窥测未来的，但是过去我们是知道的，所以我们应该传一个前驱节点，然后让当前的左指向过去，过去的右指向现在。然后将过去的值赋为现在，就可以继续下一次递归了。

四、前序与中序构建二叉树

力扣链接：力扣第105题:前序与中序构建二叉树

解析：

这道题的思路我们还是比较清楚的，根据前序确定根，然后再中序中确定根所处的位置，分割两个区间，然后递归即可。

但是再一些细节把握上还是需要注意的，递归戒截止的条件应该是当左右不构成区间了那么递归就该结束了。

/*** Definition for a binary tree node.* struct TreeNode {*     int val;*     TreeNode *left;*     TreeNode *right;*     TreeNode() : val(0), left(nullptr), right(nullptr) {}*     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}*     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}* };*/
class Solution {
public:TreeNode* build(vector<int>& preorder,vector<int>& inorder,int& previ,int inbegin,int inend){if(inbegin>inend){return nullptr;}TreeNode* newnode = new TreeNode(preorder[previ]);int inpos = inbegin;while(inpos<=inend){if(preorder[previ]==inorder[inpos]){break;}inpos++;}previ++;newnode->left = build(preorder,inorder,previ,inbegin,inpos-1);newnode->right = build(preorder,inorder,previ,inpos+1,inend);return newnode;}TreeNode* buildTree(vector<int>& preorder, vector<int>& inorder) {int previ = 0;return build(preorder,inorder,previ,0,inorder.size()-1);}
};

五、中序与后序构建二叉树

力扣链接：力扣第106题：中序与后序构建二叉树

解析：

这道题与前面的题基本上是一样的。后序与前序的不同就是我们创建好结点以后，就得先创建右子树了，才能创建左子树。这是因为后序是左子树，右子树，根这种遍历方式的，那么越靠后就先是右子树。

/*** Definition for a binary tree node.* struct TreeNode {*     int val;*     TreeNode *left;*     TreeNode *right;*     TreeNode() : val(0), left(nullptr), right(nullptr) {}*     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}*     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}* };*/
class Solution {
public:TreeNode* build(vector<int>& inorder,vector<int>& postorder,int& posti,int inbegin,int inend){if(inbegin>inend){return nullptr;}TreeNode* newnode = new TreeNode(postorder[posti]);int inpos = inbegin;while(inpos<inend){if(postorder[posti]==inorder[inpos]){break;}inpos++;}posti--;newnode->right = build(inorder,postorder,posti,inpos+1,inend);newnode->left = build(inorder,postorder,posti,inbegin,inpos-1);return newnode;}TreeNode* buildTree(vector<int>& inorder, vector<int>& postorder) {int posti = postorder.size()-1;return build(inorder,postorder,posti,0,inorder.size()-1);}
};