有权图的最短路径算法

目录

单源最短路径问题

Dijkstra算法

原理 ​

获得最短路径长度的Dijkstra代码实现

时间复杂度

算法优化

优先队列优化后的代码实现

时间复杂度

可以具体获得最短路径的Dijkstra代码实现 

Bellman-Ford算法

原理

代码实现 

Floyed算法

原理

代码实现 

单源最短路径问题

我们的起始点是固定点，从起始点出发到达其他各顶点的最短路径。

Dijkstra算法

此算法不能处理负权边，由于大量的应用不依赖负权边，所以这个算法有非常广泛的应用。

原理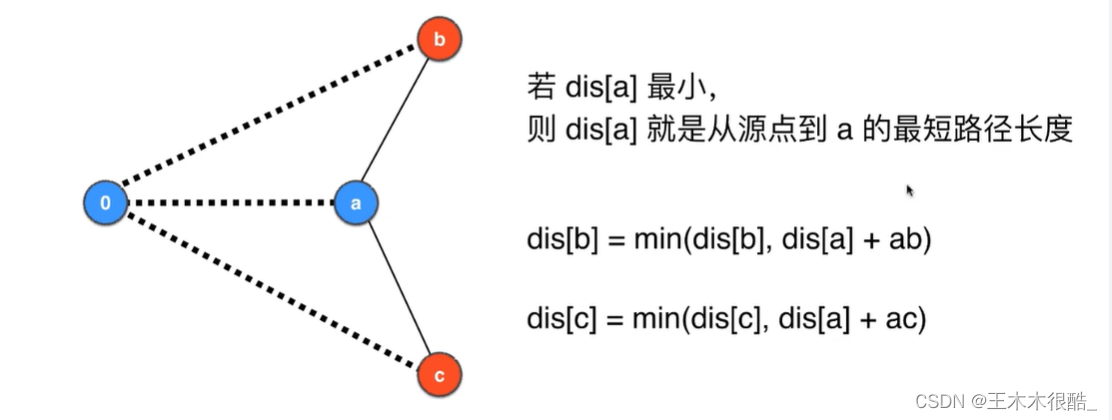 

获得最短路径长度的Dijkstra代码实现

import java.util.Arrays;public class Dijkstra {private WeightedGraph G;private int s;//源点sprivate int[] dis;//整型数组表示源点s到某个顶点的距离private boolean[] visited;//找到还没确定最短距离的顶点public Dijkstra(WeightedGraph G, int s){this.G = G;G.validateVertex(s);//验证合法性this.s = s;dis = new int[G.V()];Arrays.fill(dis, Integer.MAX_VALUE);//赋初值dis[s] = 0;//赋初值为0visited = new boolean[G.V()];while(true){//循环的第一轮找到的必是源点sint cur = -1;//最小的dis值对应的顶点是谁 int curdis = Integer.MAX_VALUE;//当前找到的最小的dis值for(int v = 0; v < G.V(); v ++)if(!visited[v] && dis[v] < curdis){curdis = dis[v];cur = v;}if(cur == -1) break;//代表当前所有的顶点都访问过了，可以退出咯visited[cur] = true;//哪些顶点的dis值已经求出来了for(int w: G.adj(cur))if(!visited[w]){if(dis[cur] + G.getWeight(cur, w) < dis[w])dis[w] = dis[cur] + G.getWeight(cur, w);}}}public boolean isConnectedTo(int v){//判断顶点和源点的连通性G.validateVertex(v);return visited[v];}public int distTo(int v){//从源点s到顶点v对应的最短路径的长度G.validateVertex(v);return dis[v];}static public void main(String[] args){WeightedGraph g = new WeightedGraph("g.txt");Dijkstra dij = new Dijkstra(g, 0);for(int v = 0; v < g.V(); v ++)System.out.print(dij.distTo(v) + " ");System.out.println();}
}

时间复杂度

算法优化

我们可以用优先队列获得v这个顶点对应的dis值，不再是v这个顶点序号的最小值了。我们的优先队列取出来的是顶点的序号，但比较起来是比较的dis值。

优先队列优化后的代码实现

import java.util.Arrays;
import java.util.PriorityQueue;public class Dijkstra {private WeightedGraph G;private int s;private int[] dis;private boolean[] visited;private class Node implements Comparable<Node>{public int v, dis;public Node(int v, int dis){this.v = v;this.dis = dis;}@Overridepublic int compareTo(Node another){return dis - another.dis;}}public Dijkstra(WeightedGraph G, int s){this.G = G;G.validateVertex(s);this.s = s;dis = new int[G.V()];Arrays.fill(dis, Integer.MAX_VALUE);dis[s] = 0;visited = new boolean[G.V()];PriorityQueue<Node> pq = new PriorityQueue<Node>();pq.add(new Node(s, 0));while(!pq.isEmpty()){int cur = pq.remove().v;if(visited[cur]) continue;visited[cur] = true;for(int w: G.adj(cur))if(!visited[w]){if(dis[cur] + G.getWeight(cur, w) < dis[w]){dis[w] = dis[cur] + G.getWeight(cur, w);pq.add(new Node(w, dis[w]));}}}}public boolean isConnectedTo(int v){G.validateVertex(v);return visited[v];}public int distTo(int v){G.validateVertex(v);return dis[v];}static public void main(String[] args){WeightedGraph g = new WeightedGraph("g.txt");Dijkstra dij = new Dijkstra(g, 0);for(int v = 0; v < g.V(); v ++)System.out.print(dij.distTo(v) + " ");System.out.println();}
}

时间复杂度

可以具体获得最短路径的Dijkstra代码实现 

import java.util.ArrayList;
import java.util.Arrays;
import java.util.Collections;
import java.util.PriorityQueue;public class Dijkstra {private WeightedGraph G;private int s;private int[] dis;private boolean[] visited;private int[] pre;private class Node implements Comparable<Node>{public int v, dis;public Node(int v, int dis){this.v = v;this.dis = dis;}@Overridepublic int compareTo(Node another){return dis - another.dis;}}public Dijkstra(WeightedGraph G, int s){this.G = G;G.validateVertex(s);this.s = s;dis = new int[G.V()];Arrays.fill(dis, Integer.MAX_VALUE);pre = new int[G.V()];Arrays.fill(pre, -1);dis[s] = 0;pre[s] = s;visited = new boolean[G.V()];PriorityQueue<Node> pq = new PriorityQueue<Node>();pq.add(new Node(s, 0));while(!pq.isEmpty()){int cur = pq.remove().v;if(visited[cur]) continue;visited[cur] = true;for(int w: G.adj(cur))if(!visited[w]){if(dis[cur] + G.getWeight(cur, w) < dis[w]){dis[w] = dis[cur] + G.getWeight(cur, w);pq.add(new Node(w, dis[w]));pre[w] = cur;}}}}public boolean isConnectedTo(int v){G.validateVertex(v);return visited[v];}public int distTo(int v){G.validateVertex(v);return dis[v];}public Iterable<Integer> path(int t){ArrayList<Integer> res = new ArrayList<>();if(!isConnectedTo(t)) return res;int cur = t;while(cur != s){res.add(cur);cur = pre[cur];}res.add(s);Collections.reverse(res);return res;}static public void main(String[] args){WeightedGraph g = new WeightedGraph("g.txt");Dijkstra dij = new Dijkstra(g, 0);for(int v = 0; v < g.V(); v ++)System.out.print(dij.distTo(v) + " ");System.out.println();System.out.println(dij.path(3));}
}

Bellman-Ford算法

原理

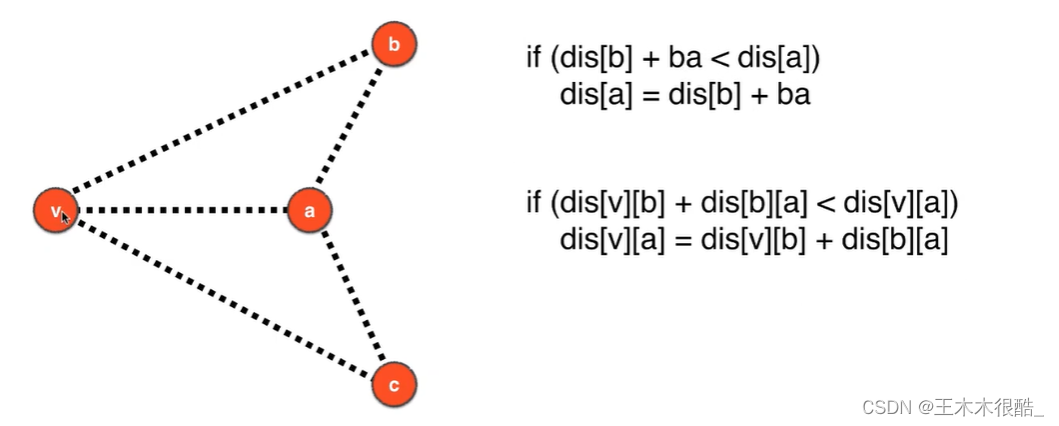
松弛操作有方向性，相当于拐个弯到达某个端点是不是比直接到达某个端点更近。此算法在有向图无向图也成立。

 

代码实现 

import java.util.Arrays;public class BellmanFord {private WeightedGraph G;private int s;private int[] dis;private boolean hasNegCycle = false;public BellmanFord(WeightedGraph G, int s){this.G = G;G.validateVertex(s);this.s = s;dis = new int[G.V()];Arrays.fill(dis, Integer.MAX_VALUE);dis[s] = 0;for(int pass = 1; pass < G.V(); pass ++){for(int v = 0; v < G.V(); v ++)for(int w: G.adj(v))if(dis[v] != Integer.MAX_VALUE &&dis[v] + G.getWeight(v, w) < dis[w])dis[w] = dis[v] + G.getWeight(v, w);}for(int v = 0; v < G.V(); v ++)for(int w : G.adj(v))if(dis[v] != Integer.MAX_VALUE &&dis[v] + G.getWeight(v, w) < dis[w])hasNegCycle = true;}public boolean hasNegativeCycle(){return hasNegCycle;}public boolean isConnectedTo(int v){G.validateVertex(v);return dis[v] != Integer.MAX_VALUE;}public int distTo(int v){G.validateVertex(v);if(hasNegCycle) throw new RuntimeException("exist negative cycle.");return dis[v];}static public void main(String[] args){WeightedGraph g = new WeightedGraph("g.txt");BellmanFord bf = new BellmanFord(g, 0);if(!bf.hasNegativeCycle()){for(int v = 0; v < g.V(); v ++)System.out.print(bf.distTo(v) + " ");System.out.println();}elseSystem.out.println("exist negative cycle.");WeightedGraph g2 = new WeightedGraph("g2.txt");BellmanFord bf2 = new BellmanFord(g2, 0);if(!bf2.hasNegativeCycle()){for(int v = 0; v < g2.V(); v ++)System.out.print(bf2.distTo(v) + " ");System.out.println();}elseSystem.out.println("exist negative cycle.");}
}

Floyed算法

原理

可以包含负权边，也可以包含负权环。 

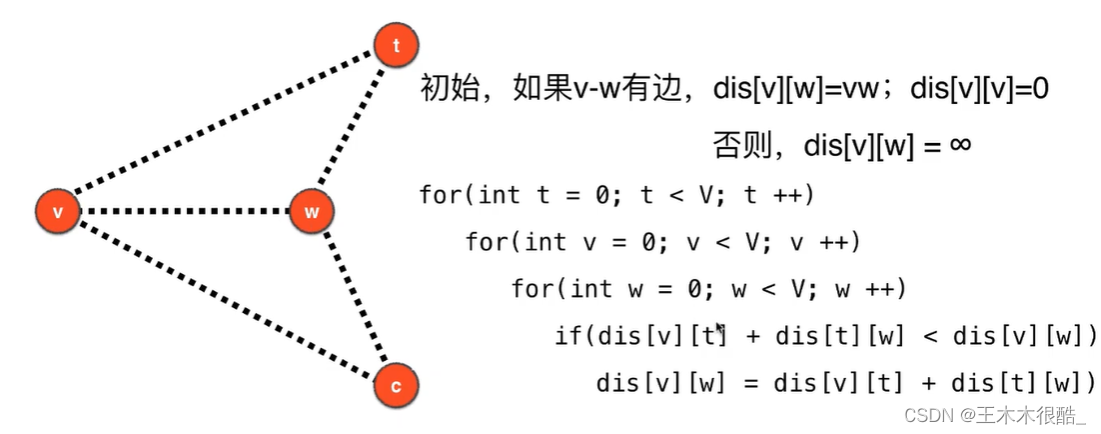
代码实现 

import java.util.Arrays;public class Floyed {private WeightedGraph G;private int[][] dis;private boolean hasNegCycle = false;public Floyed(WeightedGraph G){this.G = G;dis = new int[G.V()][G.V()];for(int v = 0; v < G.V(); v ++)Arrays.fill(dis[v], Integer.MAX_VALUE);for(int v = 0; v < G.V(); v ++){dis[v][v] = 0;for(int w: G.adj(v))dis[v][w] = G.getWeight(v, w);}for(int t = 0; t < G.V(); t ++)for(int v = 0; v < G.V(); v ++)for(int w = 0; w < G.V(); w ++)if(dis[v][t] != Integer.MAX_VALUE && dis[t][w] != Integer.MAX_VALUE&& dis[v][t] + dis[t][w] < dis[v][w])dis[v][w] = dis[v][t] + dis[t][w];for(int v = 0; v < G.V(); v ++)if(dis[v][v] < 0)hasNegCycle = true;}public boolean hasNegativeCycle(){return hasNegCycle;}public boolean isConnectedTo(int v, int w){G.validateVertex(v);G.validateVertex(w);return dis[v][w] != Integer.MAX_VALUE;}public int distTo(int v, int w){G.validateVertex(v);G.validateVertex(w);return dis[v][w];}static public void main(String[] args){WeightedGraph g = new WeightedGraph("g.txt");Floyed floyed = new Floyed(g);if(!floyed.hasNegativeCycle()){for(int v = 0; v < g.V(); v ++){for(int w = 0; w < g.V(); w ++)System.out.print(floyed.distTo(v, w) + " ");System.out.println();}}elseSystem.out.println("exist negative cycle.");WeightedGraph g2 = new WeightedGraph("g2.txt");Floyed floyed2 = new Floyed(g2);if(!floyed2.hasNegativeCycle()){for(int v = 0; v < g.V(); v ++){for(int w = 0; w < g.V(); w ++)System.out.print(floyed2.distTo(v, w) + " ");System.out.println();}}elseSystem.out.println("exist negative cycle.");}
}

本文来自互联网用户投稿，该文观点仅代表作者本人，不代表本站立场。本站仅提供信息存储空间服务，不拥有所有权，不承担相关法律责任。如若转载，请注明出处：http://www.hqwc.cn/news/226368.html

如若内容造成侵权/违法违规/事实不符，请联系编程知识网进行投诉反馈email:809451989@qq.com，一经查实，立即删除！

相关文章

http项目遇到的问题

http项目遇到的问题

问题 启动 ./httpServer 8081后, 在浏览器地址栏输入:http://192.168.186.128:8081/test_cgi&#xff0c;按下回车 陆续获取几个新连接&#xff0c; 在第四次获取到新连接时&#xff0c;输出 get a new link ... sock fd : 5 ReadLine函数 -> recv返回值为0 请求状态行的长…
阅读更多...
JRT对历史表和$get实现

JRT对历史表和$get实现

由于Cache没有什么表数据大了查询和插入性能下降的问题&#xff0c;而关系库在数据量上千万后会性能下降&#xff0c;之前关注点都是Java业务脚本化和开发部署简单&#xff0c;还没管关系库单表大问题和级联查询复杂后慢的问题&#xff0c;现在开始解决这俩问题&#xff0c;这是…
阅读更多...
【Java】7. 类型转换和类型判断

【Java】7. 类型转换和类型判断

7. 类型转换 7.1 基本类型转换 顺箭头&#xff1a;隐式转换&#xff08;自动&#xff09; 逆箭头&#xff1a;强制转换&#xff08;可能造成精度丢失&#xff09; byte a 10; int b a; int c 1000; byte d (byte) c; System.out.println(d); // -24 7.2 包装类型与基…
阅读更多...
什么是 dropblock

什么是 dropblock

大家好啊&#xff0c;我是董董灿。 之前介绍过 dropout 算法&#xff0c;它在训练神经网络中&#xff0c;可以随机丢弃神经元&#xff0c;是一种防止网络过拟合的方法。 但是在卷积神经网络中&#xff0c;dropout 的表现却不是很好&#xff0c;于是研究人员又搞了一个“结构化…
阅读更多...
第二十章总结

第二十章总结

线程简介 Windows操作系统是多任务操作系统&#xff0c;它以进程为单位。一个进程是一个包含有自身地址的程序&#xff0c;每个独立执行的程序都称为进程。也就是说每个正在执行的程序都是一个进程。系统可以分配给每一个进程有一段有限的使用CPU的时间&#xff08;也可以称为…
阅读更多...
建筑木模板厂家批发

建筑木模板厂家批发

在建筑施工中&#xff0c;木模板是一种常见且重要的施工材料&#xff0c;用于搭建混凝土浇筑的支撑结构。选择合适的建筑木模板厂家进行批发&#xff0c;对于施工质量和效率至关重要。本文将介绍建筑木模板厂家批发的重要性&#xff0c;并推荐贵港市能强优品木业作为专业的建筑…
阅读更多...
【C++初阶】四、类和对象（构造函数、析构函数、拷贝构造函数、赋值运算符重载函数）

【C++初阶】四、类和对象（构造函数、析构函数、拷贝构造函数、赋值运算符重载函数）

相关代码gitee自取&#xff1a; C语言学习日记: 加油努力 (gitee.com) 接上期&#xff1a; 【C初阶】三、类和对象 &#xff08;面向过程、class类、类的访问限定符和封装、类的实例化、类对象模型、this指针&#xff09; -CSDN博客 引入&#xff1a;类的六个默认成员函数…
阅读更多...
如何获取高质量的静态住宅IP代理？常见误区与注意事项

如何获取高质量的静态住宅IP代理？常见误区与注意事项

静态住宅IP代理在今天的网络营销领域扮演着至关重要的角色&#xff0c;静态住宅IP代理以其稳定性和高匿名性&#xff0c;为互联网业务提供了一个安全的执行环境。通过模拟真实用户的网络行为&#xff0c;这些IP代理降低了企业在网络营销活动中被识别和封禁的风险。它保护了企业…
阅读更多...
基于SpringBoot的手机官方商城系统

基于SpringBoot的手机官方商城系统

基于SpringBoot的手机官方商城系统 摘要&#xff1a;随着电子商务的发展&#xff0c;网上购物已成为人们普遍的购物方式。与此同时&#xff0c;网上支付也得到了迅速的发展&#xff0c;大有赶超传统支付的趋势。在今天这个信息化程度高、生活节奏快的现代社会&#xff0c;传统…
阅读更多...
Airtest自动化测试工具实战演练

Airtest自动化测试工具实战演练

一开始知道Airtest大概是在年初的时候&#xff0c;当时&#xff0c;看了一下官方的文档&#xff0c;大概是类似Sikuli的一个工具&#xff0c;主要用来做游戏自动化的&#xff0c;通过截图的方式用来解决游戏自动化测试的难题。最近&#xff0c;移动端测试的同事尝试用它的poco库…
阅读更多...
Centos7安装配置nginx

Centos7安装配置nginx

快捷查看指令 ctrlf 进行搜索会直接定位到需要的知识点和命令讲解&#xff08;如有不正确的地方欢迎各位小伙伴在评论区提意见&#xff0c;小编会及时修改&#xff09; Centos7安装配置nginx Nginx介绍 Nginx (engine x) 是一个高性能的 HTTP 和 反向代理 服务&#xff0c;也…
阅读更多...
浅谈硬件连通性测试几大优势

浅谈硬件连通性测试几大优势

硬件连通性测试是确保硬件系统正常运行、提高系统可靠性和降低生产成本的关键步骤。在现代工程和制造中&#xff0c;将连通性测试纳入生产流程是一个明智的选择&#xff0c;有助于确保硬件产品的质量和性能达到最优水平。本文将介绍硬件连通性测试的主要优势有哪些! 一、提高系…
阅读更多...
推荐文章
最新文章

Copyright @ 2022~2023