期权二叉树估值与图计算-编程知识

期权二叉树估值与图计算

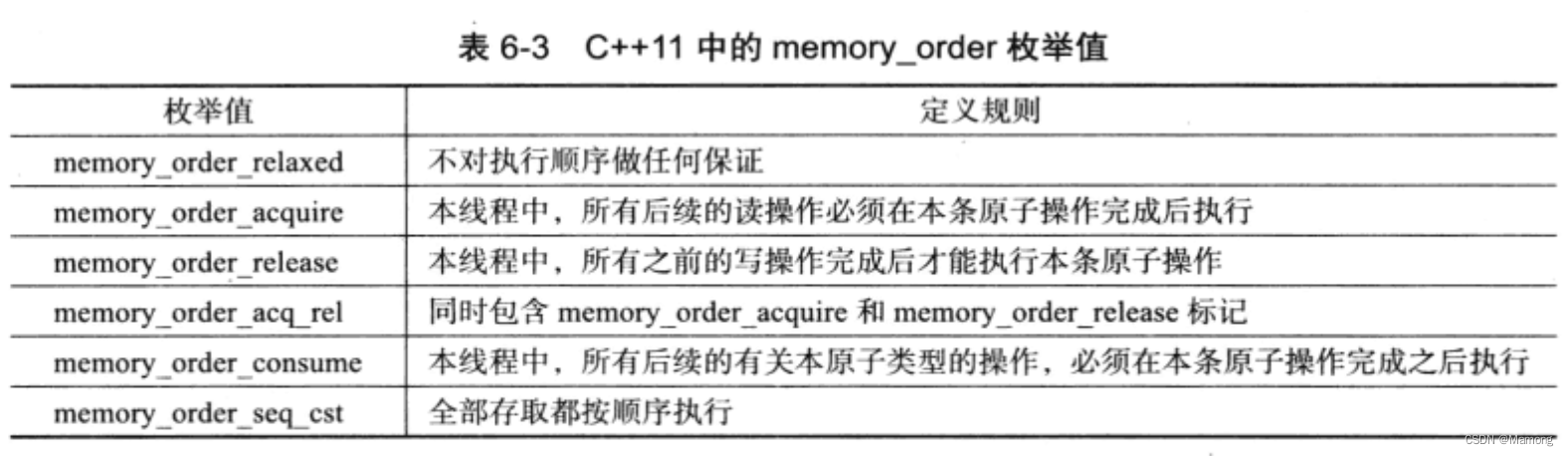
传统期权二叉树的算法都是基于数组的，对于没有编程基础的人来说非常不直观。二叉树是一种特殊的图，可以用python networkx这个图算法库实现，这个库不仅包含常用的图算法，还包含简单的绘图功能，非常适合研究分析使用。

def binarytree_europtionfull(S,K,r,q,sigma,t,steps,op_type):u=np.exp(sigma*np.sqrt(t/steps))d=1/uP=(np.exp((r-q)*t/steps)-d)/(u-d)#二叉完全树    G=nx.full_rary_tree(2,2**(steps+1)-1)G.nodes[0]['price']=S#正推过程for (parent,children) in nx.bfs_successors(G,0):#print(parent,children)G.nodes[children[0]]['price']=G.nodes[parent]['price']*d;G.nodes[children[1]]['price']=G.nodes[parent]['price']*u;#对最后一天到期日计算所有情况下的payofffor i in nx.descendants_at_distance(G,0,steps):G.nodes[i]['opt_val']=np.maximum(G.nodes[i]['price']-K,0)#倒推过程for (parent,children) in reversed(list(nx.bfs_successors(G,0))):G.nodes[parent]['opt_val']=((1-P)*G.nodes[children[0]]['opt_val']+(P)*G.nodes[children[1]]['opt_val'])*np.exp(-r*t/steps)    #print(node)dat=G.nodes.data()#生成图形显示，可注释掉。pos = graphviz_layout(G, prog="dot")nx.draw(G, pos, labels={k:"price: %.2f\n opt_val:%.2f"%(v['price'],v['opt_val']) for (k,v) in dat},with_labels=True)plt.show()return G.nodes[0]['opt_val']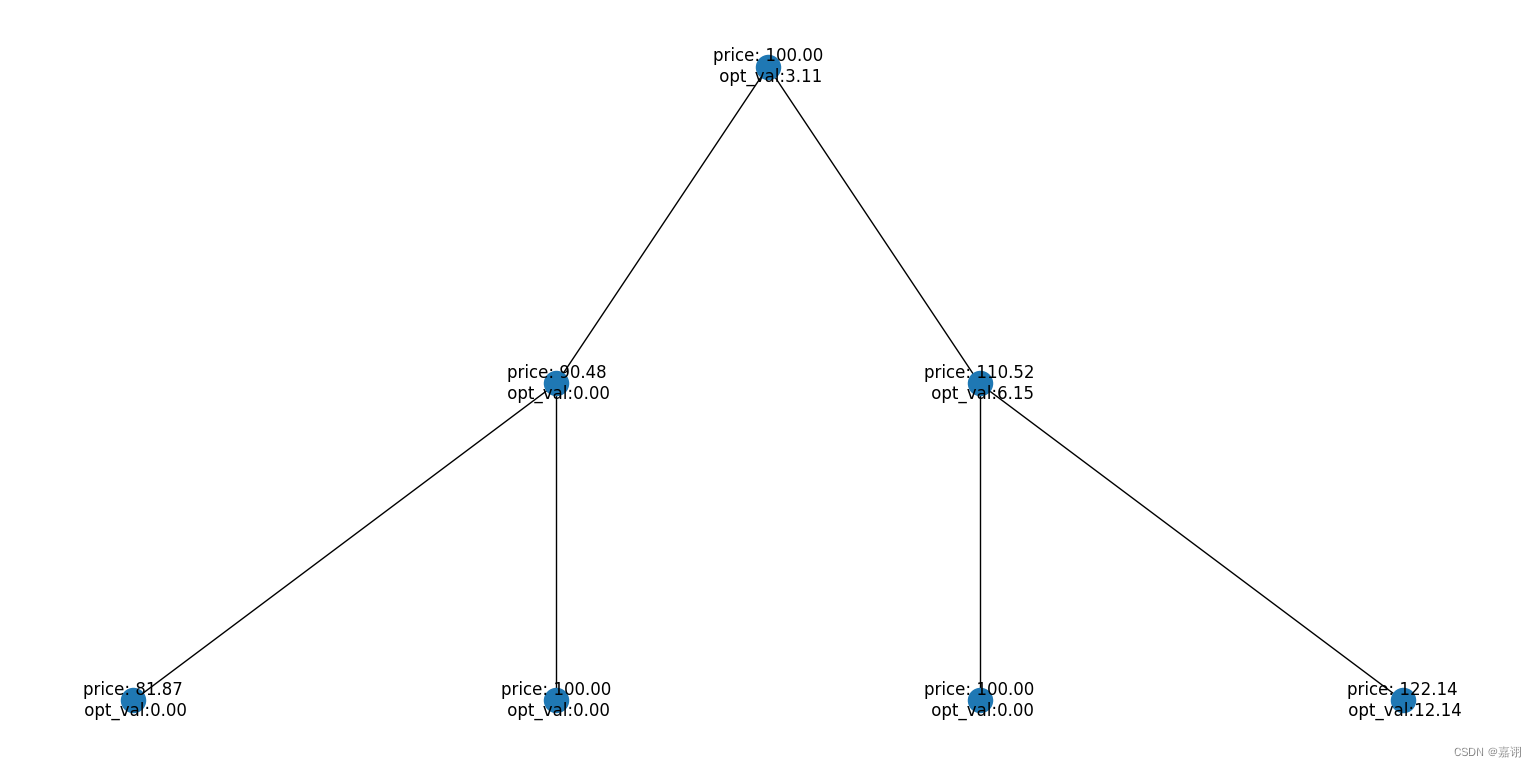

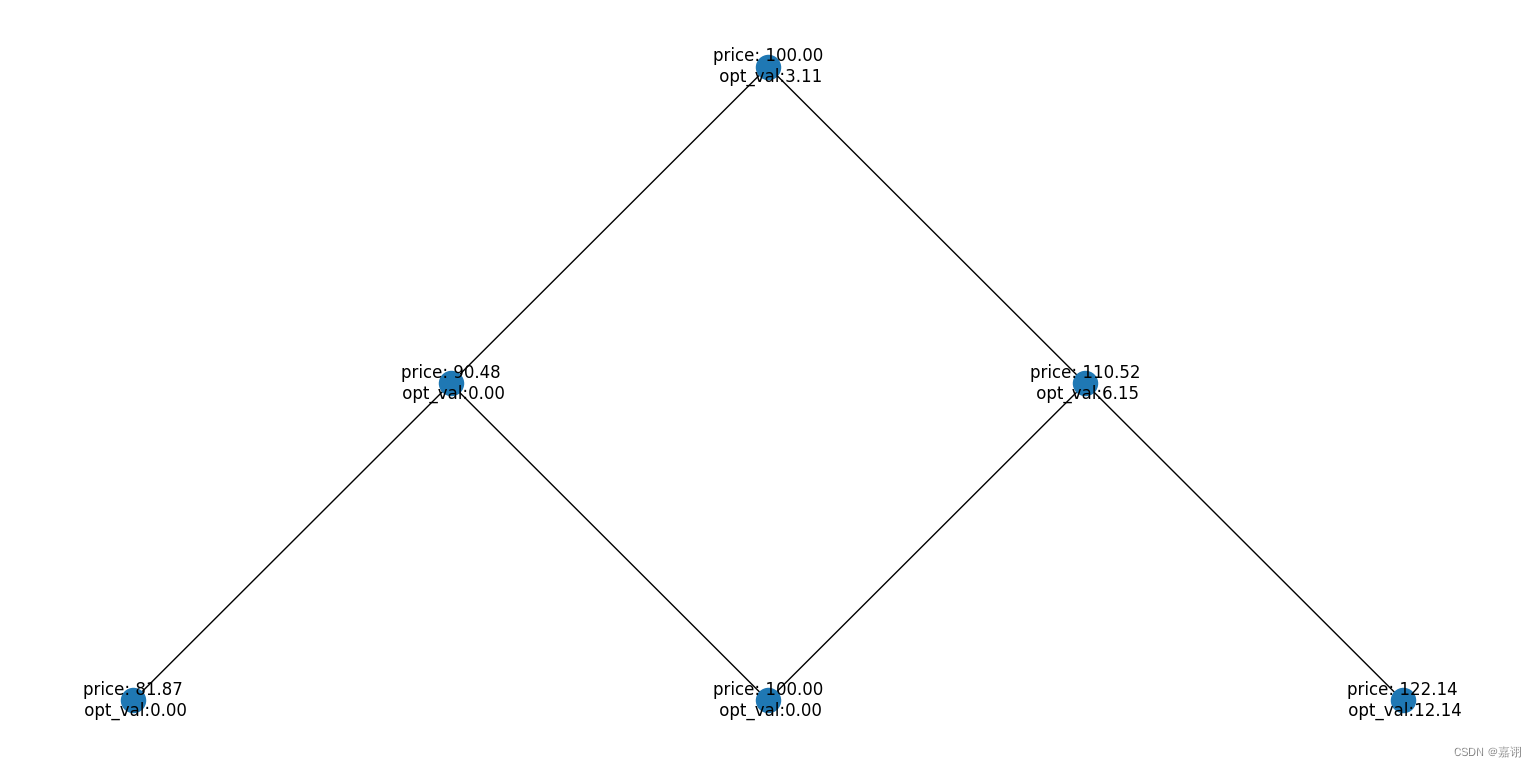
直接用二叉树空间复杂度为 $2^{n}$ ，呈指数增长。我们注意到，对于中间的节点，其实有一些是相等的，因为对于一支标的价格，S*u*d=S*d*u，所以我们可以将这些节点合并，这就是网格模式。

def binarytree_europtionLattice(S,K,r,q,sigma,t,steps,op_type):u=np.exp(sigma*np.sqrt(t/steps))d=1/uP=(np.exp((r-q)*t/steps)-d)/(u-d)G = nx.Graph()G.add_node(0)G.nodes[0]['price']=Scnt=0for i in range(steps):for j in range(i+1):#print(j,i,cnt,cnt+i+1,cnt+i+2)G.add_edge(cnt,cnt+i+1)G.add_edge(cnt,cnt+i+2)cnt+=1#正推过程for (parent,children) in nx.bfs_successors(G,0):if len(children)==2:G.nodes[children[0]]['price']=G.nodes[parent]['price']*d;G.nodes[children[-1]]['price']=G.nodes[parent]['price']*u;#对最后一天到期日计算所有情况下的payoff，for i in nx.descendants_at_distance(G,0,steps):G.nodes[i]['opt_val']=np.maximum(G.nodes[i]['price']-K,0)#倒推过程for (parent,children) in reversed(list(nx.bfs_successors(G,0))):G.nodes[parent]['opt_val']=((1-P)*G.nodes[children[-1]-1]['opt_val']+(P)*G.nodes[children[-1]]['opt_val'])*np.exp(-r*t/steps)    #生成图形显示，可注释掉。pos = graphviz_layout(G, prog="dot")nx.draw(G, pos, labels={k:"price: %.2f\n opt_val:%.2f"%(v['price'],v['opt_val']) for (k,v) in dat},with_labels=True)plt.show()return G.nodes[0]['opt_val']