均匀与准均匀 B样条算法-编程知识

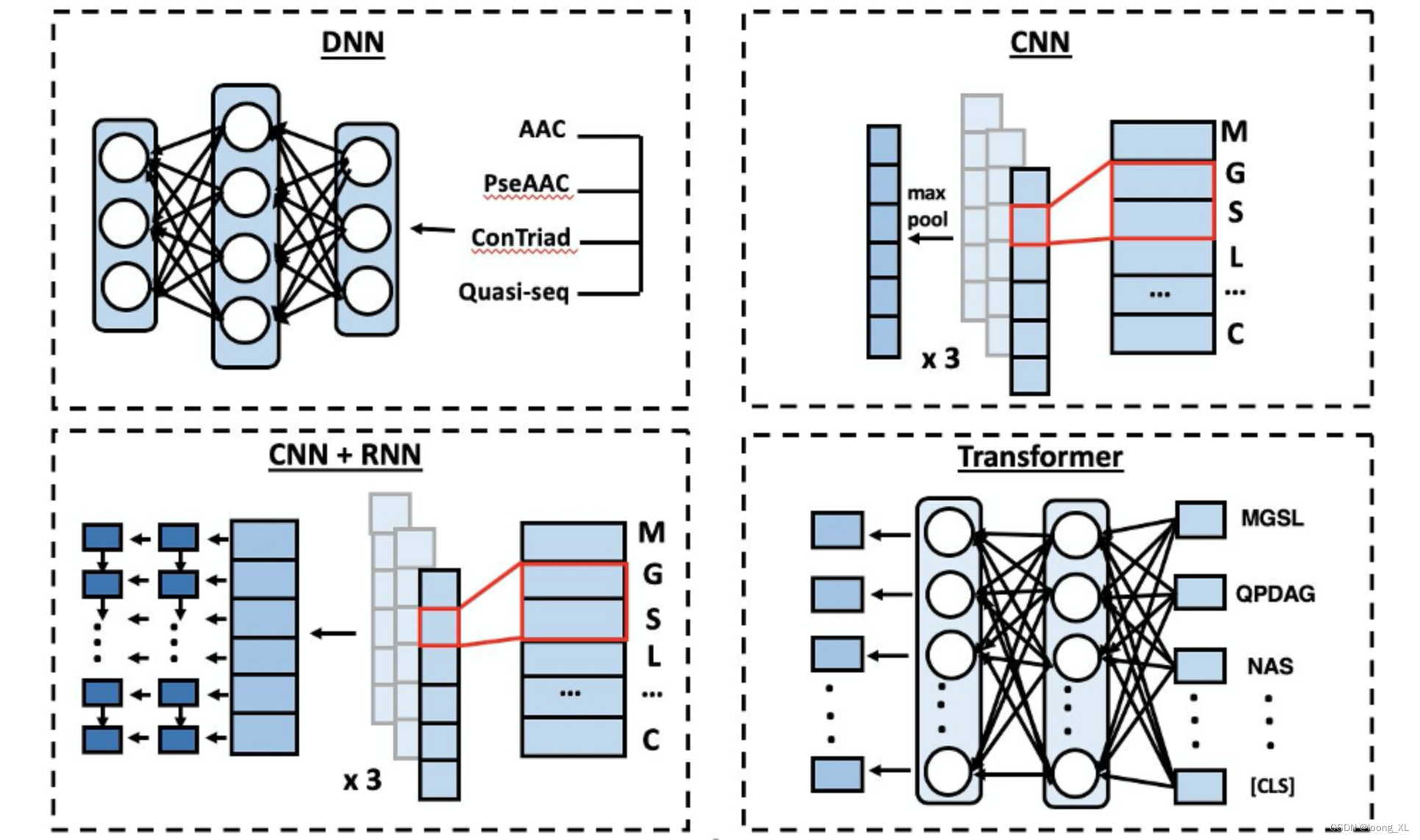
B 样条曲线的定义

$\sum_{i=0}{n} P_i F_{i, k}(t)$

方程中 $n + 1$ 个控制点， $P_i$ , $\cdots n$ 要用到 $n + 1$ 个 $k$ 次 B 样条基函数 $F_{i, k}$ , $\cdots, n$ , 节点矢量为 $[t_0, t_1, \cdots, t_{n+k+1}]$ 。 $F_{i, k}(t)$ 是由一个称为节点矢量的非递减的参数 $t$ 的序列， $t_0 \leq t_1 \leq \cdots \leq t_{n+k+1}$ 所决定的 $k$ 次分段多项式。

B 样条曲线划分为四种类型，均匀 B 样条曲线，准均匀B 样条曲线，分段 Bezier 曲线和非均匀 B 样条曲线。

定义域

给定 $n + 1$ 个控制点， $P_i$ , $\cdots n$ , 相应地要求 $n + 1$ 个 B 样条基函数 $F_{i, k}(t)$ 定义一个 $k$ 次 B 样条曲线，这 $n + 1$ 个 $k$ 次 B 样条由节点矢量 $[t_0, t_1, \cdots t_{n+k+1}]$ 所决定。
并非这个些节点矢量所包含的 $n + k + 1$ 个区间都在该曲线的定义域，其中两端的各 $k$ 个几点区间，不能作为 B 样条曲线的定义区间。
这是因为 $n + 1$ 个控制点中最前的 $n + 1$ 个顶点 $P_i$ , $\cdots k$ 定义了 B 样条曲线的首段，其定义区间为 $t\in [t_k, t_{k+1}]$ 往后移动一个顶点 $P_i$ ， $\cdots k+1$ 定义第二段，其定义区间为 $\in [t_{k+}, t_{k+2}]$ 依次类推，最后 $k + 1$ 个顶点， $P_i$ , $\cdots n$ 定义最后一段，其定义区间为 $t\in[t_n, t_{n+1}]$ , 因此，高于零次的 $k$ 次B 样条曲线的定义域为

$\in [t_k, t_{n+1}]$

三次均匀 B 样条曲线

$\begin{cases} F_{0,3}(t) = \frac{1}{6} (1-t)^3 = (-t^3 + 3t^2 -3t+1)\\ F_{1,3}(t) = \frac{1}{6} (3t^3 - 6t^2 +4)\\ F_{2,3}(t) = \frac{1}{6} (-3t^3 + 3t^2 + 3t +1) \\ F_{3,3}(t) = \frac{1}{6} t^3\\ \end{cases}$

在这里插入图片描述

三次 B 样条的几何性质

$\begin{cases} p(0) = \frac{1}{6}(p_0 + 4 p_1 + p_2) = \frac{1}{3} (\frac{p_0 + p_2}{2}) + \frac{2}{3}p_1 = \frac{1}{3}p_m + \frac{2}{3}p_1\\ p(1) = \frac{1}{6}(p_1 + 4 p_2 + p_3) = \frac{1}{3} (\frac{p_1 + p_3}{2}) + \frac{2}{3}p_2 = \frac{1}{3}p_n + \frac{2}{3}p_2\\ \end{cases}$

$\begin{cases} p'(0) = \frac{1}{2}(p_2 - p_0) \\ p'(1) = \frac{1}{2}(p_3 + p_1) \\ \end{cases}$

$\begin{cases} p''(0) = p_0 - 2p_1 + p_2 = 2(\frac{p_0 + p_2}{2} -p)= 2(p_m - p_1) \\ p''(1) = p_1 - 2p_2 + p_3 = 2(\frac{p_1 + p_3}{2} -p_2)= 2(p_n - p_2) \\ \end{cases}$

在这里插入图片描述

#include <QWidget>
#include <QApplication>
#include <QPainter>
#include <QPointF>
#include <QPainterPath>const double knot[13] = {-3/6.0, -2/6.0, -1/6.0, 0.0, 1 / 6.0, 2 / 6.0, 3 / 6.0, 4 / 6.0, 5 / 6.0, 1.0, 7/ 6.0, 8/ 6.0, 9/6.0};double BasisFunctionValue(double t, int i, int k)
{double val1, val2, val;if (k == 0){if ((t >= knot[i]) && t < knot[i + 1]){return 1.0;}else{	// 其它return 0.0;}}if (k > 0){if (t < knot[i] || t > knot[i + k + 1]) {return 0.0;		// 其它}else{double coffcient1, coffcient2;	// 凸组合系数1 凸组合系数 2double denominator = 0.0;		// 分母denominator = knot[i + k] - knot[i];if (denominator == 0.0){// 约定 0/0 = 0coffcient1 = 0.0;}else{coffcient1 = (t - knot[i]) / denominator;	// 计算的第一项}denominator = knot[i + k + 1] - knot[i + 1];	// 递推公式第二项分母if (denominator == 0.0){// 约定 0/0 = 0coffcient2 = 0.0;}else{coffcient2 = (knot[i + k + 1] - t) / denominator;	// 递推公式第二项}val1 = coffcient1 * BasisFunctionValue(t, i, k - 1);	// 递推公式第一项的只val2 = coffcient2 * BasisFunctionValue(t, i+1, k - 1);	// 递推公式第二项的只val = val1 + val2;	// 基函数的值}}return val;
}void drawBSplineCure(QPainter* painter, const std::vector<QPointF>& P)
{// Set line colorQColor lineColor(0, 0, 255);// Set point colorQColor pointColor(255, 0, 0);QPainterPath bezierPath;QPen pen(lineColor);pen.setWidth(2);  // Set the line width as neededpainter->setPen(pen);QPointF center(900, 600);  // Center coordinatesint k = 3;  // Degree of the B-spline curvefor (int i = k; i <= P.size() - k; ++i){double tStep = 0.01;for (double t = 0.0; t <= 1.0; t += tStep){QPointF p(0, 0);  // Discrete pointfor (int j = 0; j < P.size(); ++j){double BValue = BasisFunctionValue(t, j, k);p += P[j] * BValue;}if (t == 0.0){bezierPath.moveTo(p + center);}else{bezierPath.lineTo(p + center);}painter->setPen(pointColor);painter->setBrush(Qt::NoBrush);painter->drawEllipse(p + center, 5, 5);}}painter->drawPath(bezierPath);
}void drawControlPolygon(QPainter* painter, std::vector<QPointF> P)
{QColor lineColor(0, 0, 0);QColor pointColor(0, 0, 255);  // Blue color for pointsQPen polyLinePen(lineColor);painter->setPen(polyLinePen);QBrush pointBrush(pointColor);painter->setBrush(pointBrush);QPointF center(900, 600);QVector<QPointF> shiftedPoints;std::transform(P.begin(), P.end(), std::back_inserter(shiftedPoints),[center](const QPointF& point) { return point + center; });painter->drawPolyline(shiftedPoints.data(), shiftedPoints.size());for (const QPointF& point : shiftedPoints){painter->drawEllipse(point, 5, 5);}}std::vector<QPointF> getControlPoints(){std::vector<QPointF> controlPoints = {QPointF(-600, -50),QPointF(-500, 200),  // 控制点QPointF(-160, 250),QPointF(-250, -300),QPointF(160, -200),  // 控制点QPointF(200, 200),QPointF(600, 180),QPointF(700, -60),   // 控制点QPointF(500, -200)};return controlPoints;
}class MyWidget : public QWidget {
public:MyWidget(QWidget* parent = nullptr) : QWidget(parent) {setFixedSize(1800, 1200);}protected:void paintEvent(QPaintEvent* event) override {Q_UNUSED(event);QPainter painter(this);painter.setRenderHint(QPainter::Antialiasing, true);std::vector<QPointF> controlPoints = getControlPoints();drawBSplineCure(&painter, controlPoints);drawControlPolygon(&painter, controlPoints);}public:int n = 8;int k = 3;};int main(int argc, char* argv[]) {QApplication app(argc, argv);MyWidget widget;widget.show();return app.exec();
}