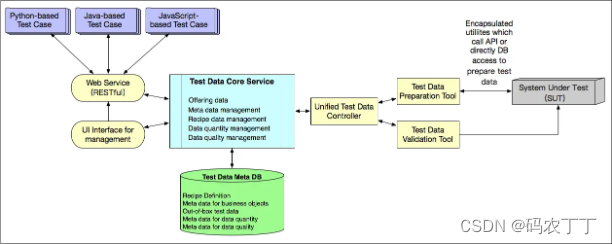
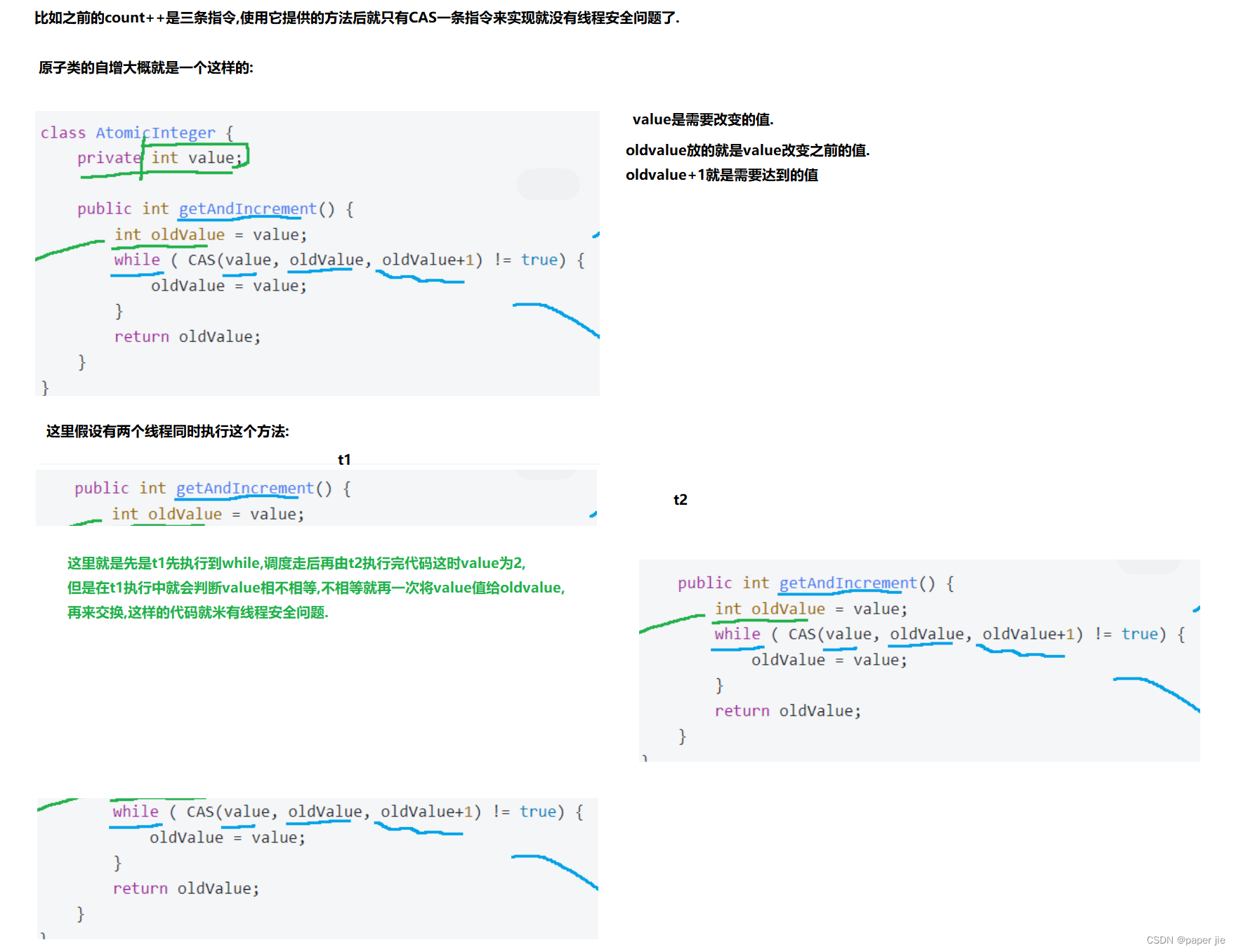
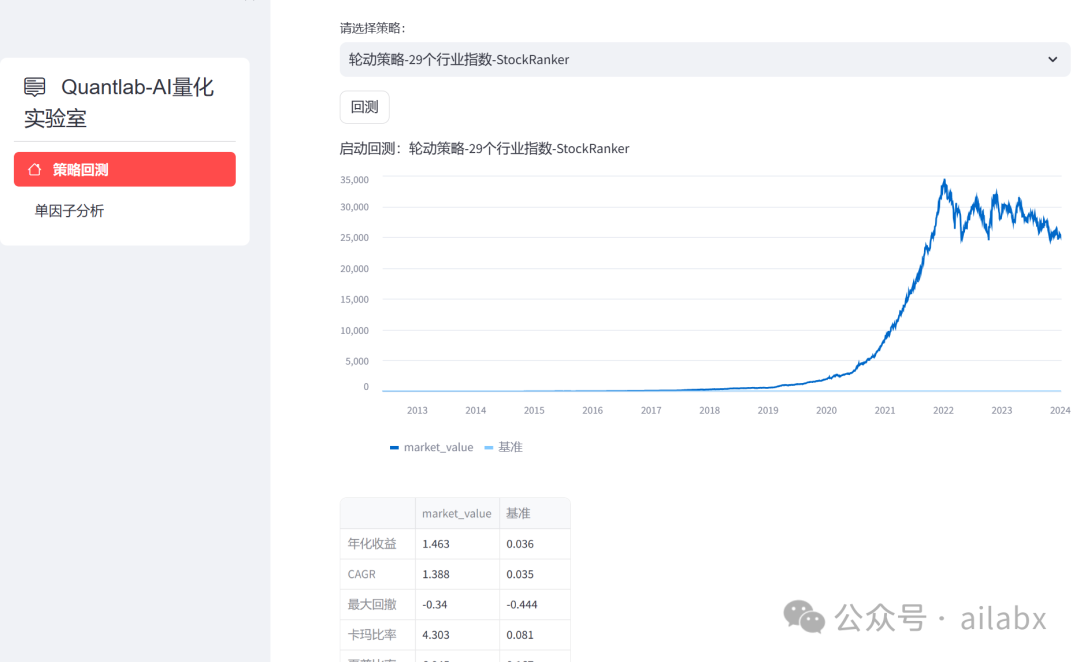
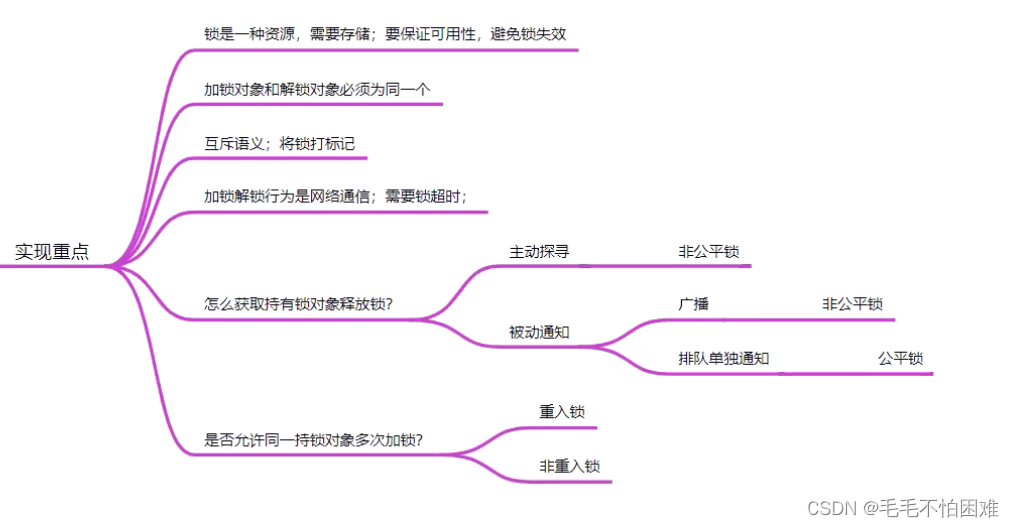
贝贝通过指令获得了 n 个木棍和  m 个钻石，以及一个工作台，他想要制造尽可能多的工具。
一共有五种钻石工具，下面是每种钻石工具的合成方案：

输入
第一行，一个整数，为数组组数 T (1 ≤ T ≤ 1e5)。
接下来的 T 行，每行两个以空格分隔的整数 n,m (0 ≤ n,m ≤ 1e9)。

输出
输出 T 行，每行一个整数，表示该组数据可制作工具数量的最大值。

Input
3
2 9
5 4
6 7

Output
2
3
4

解析1：
容易知道我们选择工具 3 和 5，由于两个需要的材料个数对称。所以设x,y。x个钻石，y根木棍和x根木棍，y个钻石所能合成的工具的个数是一样的。
假设 x>y:

当x>=2*y的时候，由于我们的比例可以按照2：1来制造，最多得到了y个工具。 

当x<2*y的时候，我们就要尽可能让由于两个方法都是按照2：1来制作。我们就用求解当前最优。我们先把x比y多的拿去制作，找到x，y相同。
即 x-2*k=y-k,解得k=x-y;
当 x，y 相同的时候，也就是制作了x-y 把工具后，这时候 x 还剩下 2*y-x 个，这时候两种材料个数相同，用这些材料按照 3:3 制作。

#include <bits/stdc++.h>
using namespace std;
#define int long long
#define endl '\n'
#define ios ios::sync_with_stdio(false),cin.tie(0),cout.tie(0);
int gcd(int a,int b) { return b? gcd(b,a%b) : a; }
typedef pair<int,int> PII;
const double PI=acos(-1.0);
const int N=2e6+10;
int x,y;
void solve()
{cin>>x>>y;if (x<y) swap(x,y);if (x>=2*y) cout<<y<<endl;else {int k=x-y;k +=(2*y-x)/3*2;if ((2*y-x)%3==2) k++;cout<<k<<endl;}
}
signed main()
{ios;int T=1;cin>>T;while (T--) solve(); return 0;
}

解析2：
x+2y=n,y+2x=m,其中x是我们造钻石铲的数量，y是我们造钻石剑的数量，解这个方程即可。
解得 x=(2*m-n)/3.0，y=(2*n-m)/3.0。 

#include <bits/stdc++.h>
using namespace std;
#define int long long
#define endl '\n'
#define ios ios::sync_with_stdio(false),cin.tie(0),cout.tie(0);
int gcd(int a,int b) { return b? gcd(b,a%b) : a; }
typedef pair<int,int> PII;
const double PI=acos(-1.0);
const int N=2e6+10;
int n,m;
void solve()
{cin>>n>>m;double x=(2*m-n)/3.0;double y=(2*n-m)/3.0;if (x<0) cout<<min(n/2,m)<<endl;else if (y<0) cout<<min(m/2,n)<<endl;else cout<<(int)(x+y)<<endl;
}
signed main()
{ios;int T=1;cin>>T;while (T--) solve(); return 0;
}