二叉树经典OJ题（2）-编程知识

一、根据二叉树创建字符串

. - 力扣（LeetCode）

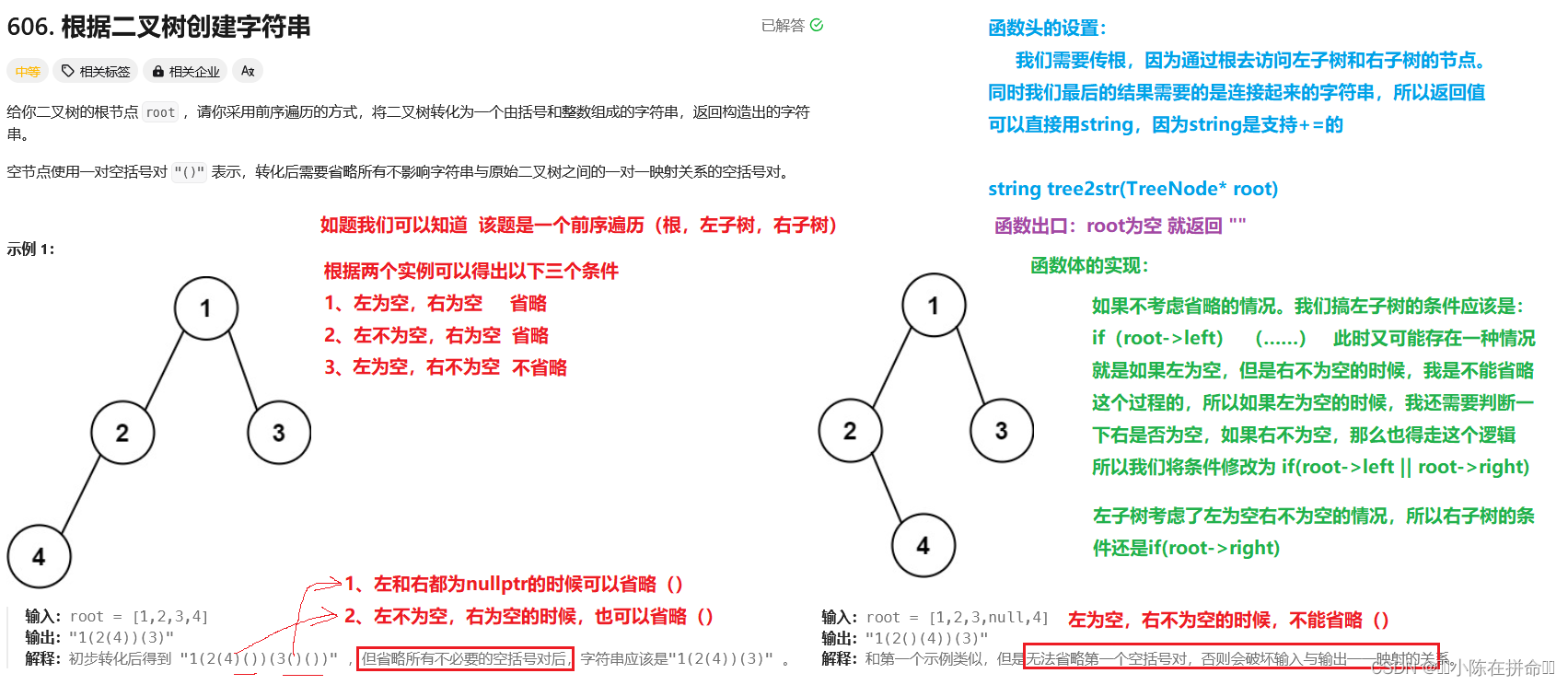
class Solution {
public://前序遍历：根 左 右//左子树为空，右子树不为空的时候，不能省略左//左不为空，右子树为空的时候，可以省略右//都为空，可以省略string tree2str(TreeNode* root) { if(root==nullptr) return "";string str=to_string(root->val);if(root->left||root->right) {str+='(';str+=tree2str(root->left);str+=')';}if(root->right){str+='(';str+=tree2str(root->right);str+=')';}return str;}};

二、二叉树的最近公共祖先

. - 力扣（LeetCode）

思路1：

//策略1 1、如果一个在我的左子树，一个在我的右子树，那么我就是最近公共祖先//      2、如果两个走在我的左，就去左找  都不在我的左，那就去我的右找//      3、如果我自己就是，那另一个必然是我的孩子 返回我自己
class Solution {
public:bool isintree(TreeNode* root, TreeNode* x){if(root==nullptr) return false;else return root==x||isintree(root->left,x)||isintree(root->right,x);}TreeNode* lowestCommonAncestor(TreeNode* root, TreeNode*&p, TreeNode*&q){if(root==nullptr) return nullptr;if(root==p||root==q) return root;//此时就要去看看我的左子树和右子树找一找bool pleft=isintree(root->left,p);bool pright=!pleft;bool qleft=isintree(root->left,q);bool qright=!qleft;if(pleft&&qright ||qleft&&pright) return root;else if(pleft&&qleft) return lowestCommonAncestor(root->left,p,q);else return lowestCommonAncestor(root->right,p,q);}
};

思路2：

class Solution {
public://策略2：利用dfs将p和q的路径存到容器中，然后转化成链表相交的问题 TreeNode* lowestCommonAncestor(TreeNode* root, TreeNode* p, TreeNode* q){if(root==p||root==q) return root;stack<TreeNode*> Ppath;stack<TreeNode*> Qpath;dfs(root,p,Ppath);//找pdfs(root,q,Qpath);//找q//此时已经找到了两条目标路径，然后让长的那一条pop直到和另一条相等while(Ppath.size()!=Qpath.size()){if(Ppath.size()>Qpath.size()) Ppath.pop();else Qpath.pop();}//此时两个一起出，直到相等就返回while(Ppath.top()!=Qpath.top()){Ppath.pop();Qpath.pop();}return Ppath.top();}bool dfs(TreeNode* root, TreeNode* x,stack<TreeNode*>&path){if(root==nullptr) return false;//此时可以入栈，然后去左边和右边找一下path.push(root);//无论如何都入if(root==x||dfs(root->left,x,path)||dfs(root->right,x,path)) return true;//左边或右边找到，返回true//两边都没有找到，说明root不是我们想要的，出栈并返回false；path.pop();//都没找到，就得回溯return false;}
};

三、二叉搜索树与双向链表

二叉搜索树与双向链表_牛客题霸_牛客网

class Solution {
public:void inorder(TreeNode* cur,TreeNode*&prev){if(cur==nullptr) return;//cur指向的就是中序遍历的结果inorder(cur->left,prev);//这里出现的cur就是中序的结果cur->left=prev;if(prev) prev->right=cur;prev=cur;inorder(cur->right,prev);}TreeNode* Convert(TreeNode* pRootOfTree){TreeNode*prev=nullptr;inorder(pRootOfTree,prev);TreeNode*head=pRootOfTree; //不断往左找，找到最链表头while(head&&head->left) head=head->left;return head;}
};

技巧：在递归过程中，我们想要有一个变量记录全过程（该题中的prev），第一种方法就是设置成全局变量，第二种方法就是传引用。

四、前序和中序遍历序列构建二叉树

. - 力扣（LeetCode）

class Solution {
public:TreeNode* _buildTree(vector<int>& preorder, vector<int>& inorder,int&pi,int begin,int end) {//先创建该节点if(begin>end) return nullptr;TreeNode*root=new TreeNode(preorder[pi]);//然后根据该节点分割左右区间 在中序数组中找到树int rooti=begin;while(rooti<=end){if(inorder[rooti]==preorder[pi]) break;++rooti;}//划分区间去左子树和右子树找++pi;root->left=_buildTree(preorder,inorder,pi,begin,rooti-1);root->right=_buildTree(preorder,inorder,pi,rooti+1,end);return root;}TreeNode* buildTree(vector<int>& preorder, vector<int>& inorder) {//前序遍历：根 左子树 右子树    一个指针去遍历前序找根//中序遍历：左子树 根 右子树    划分左右区间//前序帮我们找根，中序帮我们划分区间int pi=0;//帮助我们按顺序遍历前序数组return _buildTree(preorder,inorder,pi,0,inorder.size()-1);}
};

五、中序和后序序列遍历构建二叉树

. - 力扣（LeetCode）

class Solution {
public:TreeNode* _buildTree(vector<int>& inorder, vector<int>& postorder,int&pi,int begin,int end){if(begin>end) return nullptr;TreeNode*root=new TreeNode(postorder[pi]);//肯定要先建立根//开始划分区间int rooti=begin;while(rooti<=end) {if(inorder[rooti]==postorder[pi]) break;++rooti;}//开始延伸，先延伸右子树，再延伸左子树--pi;root->right= _buildTree(inorder,postorder,pi,rooti+1,end);root->left= _buildTree(inorder,postorder,pi,begin,rooti-1);return root;}//后序遍历 左子树 右子树 根//中序遍历 左子树 根 右子树//后序遍历帮我们找根。   中序遍历帮我们划分左右区间TreeNode* buildTree(vector<int>& inorder, vector<int>& postorder){int n=inorder.size();int pi=n-1;return _buildTree(inorder,postorder,pi,0,n-1);}
};

六、非递归实现二叉树的前序遍历

. - 力扣（LeetCode）

class Solution {
public://根 左子树 右子树//根、左子树、然后再栈里面搞右子树vector<int> preorderTraversal(TreeNode* root) {stack<TreeNode*> st;vector<int> ret;if(root==nullptr) return ret;TreeNode*cur=root;//1、访问左路节点//2、访问左路节点的右子树while(!st.empty()||cur) //cur不为空表示当前的树还没访问，栈不为空表示还有右子树没有访问{while(cur){ret.push_back(cur->val);st.push(cur);cur=cur->left;}//开始去访问右子树TreeNode* t=st.top();st.pop();//转化成子问题，去访问节点的右树cur=t->right;}return ret;}
};

七、非递归实现二叉树的中序遍历

. - 力扣（LeetCode）

class Solution {
public:
//中序  左子树 根 右子树 
//1 左路节点
//2 左子树的右路节点
//从栈里取到左路节点，意味着左路节点，以为着这个节点的左子树已经访问完了。
// 非递归实现 vector<int> inorderTraversal(TreeNode* root) {stack<TreeNode*> st;vector<int> ret;if(root==nullptr) return ret;TreeNode*cur=root;while(cur||!st.empty()){while(cur){st.push(cur);cur=cur->left;}//此时，从栈中拿出来TreeNode* t=st.top();st.pop();ret.push_back(t->val);cur=t->right;}return ret;}
};

八、非递归实现二叉树的后序遍历

. - 力扣（LeetCode）

class Solution {
public:vector<int> postorderTraversal(TreeNode* root) { stack<TreeNode*> st;vector<int> ret;if(root==nullptr) return ret;TreeNode*cur=root;//1、访问左路节点//2、访问左路节点的右子树TreeNode*prev=nullptr;while(!st.empty()||cur) //cur不为空表示当前的树还没访问，栈不为空表示还有右子树没有访问{while(cur){st.push(cur);cur=cur->left;}//开始去访问右子树TreeNode* t=st.top();if(t->right==nullptr||t->right==prev){ret.push_back(t->val);st.pop();prev=t;}//从栈中弹出的节点，我们只能确定其左子树肯定访问完了，但是无法确定右子树是否访问过。else cur=t->right;}return ret;}
};

还有一种思路就是，因为我们的结果是存在一个数组去返回的，因此我们可以按照前序遍历的逻辑：将问题拆分成右路节点和右路节点的左子树。然后按照根、右子树、左子树的顺序去访问(和后序相反),最后再逆置我们的返回数组即可。这是一种取巧的方法，但是能成功是因为该题是将结果放到一个数组中返回的，如果该题是要求我们边遍历边访问，比如打印，那么该方法就不可行。

class Solution {
public:vector<int> postorderTraversal(TreeNode* root) {stack<TreeNode*> st;vector<int> ret;if(root==nullptr) return ret;TreeNode*cur=root;//1、访问右路节点//2、访问右路节点的左子树while(!st.empty()||cur) //cur不为空表示当前的树还没访问，栈不为空表示还有左子树没有访问{while(cur){ret.push_back(cur->val);st.push(cur);cur=cur->right;}//开始去访问左子树TreeNode* t=st.top();st.pop();//转化成子问题，去访问节点的右树cur=t->left;}reverse(ret.begin(),ret.end());return ret;}
};