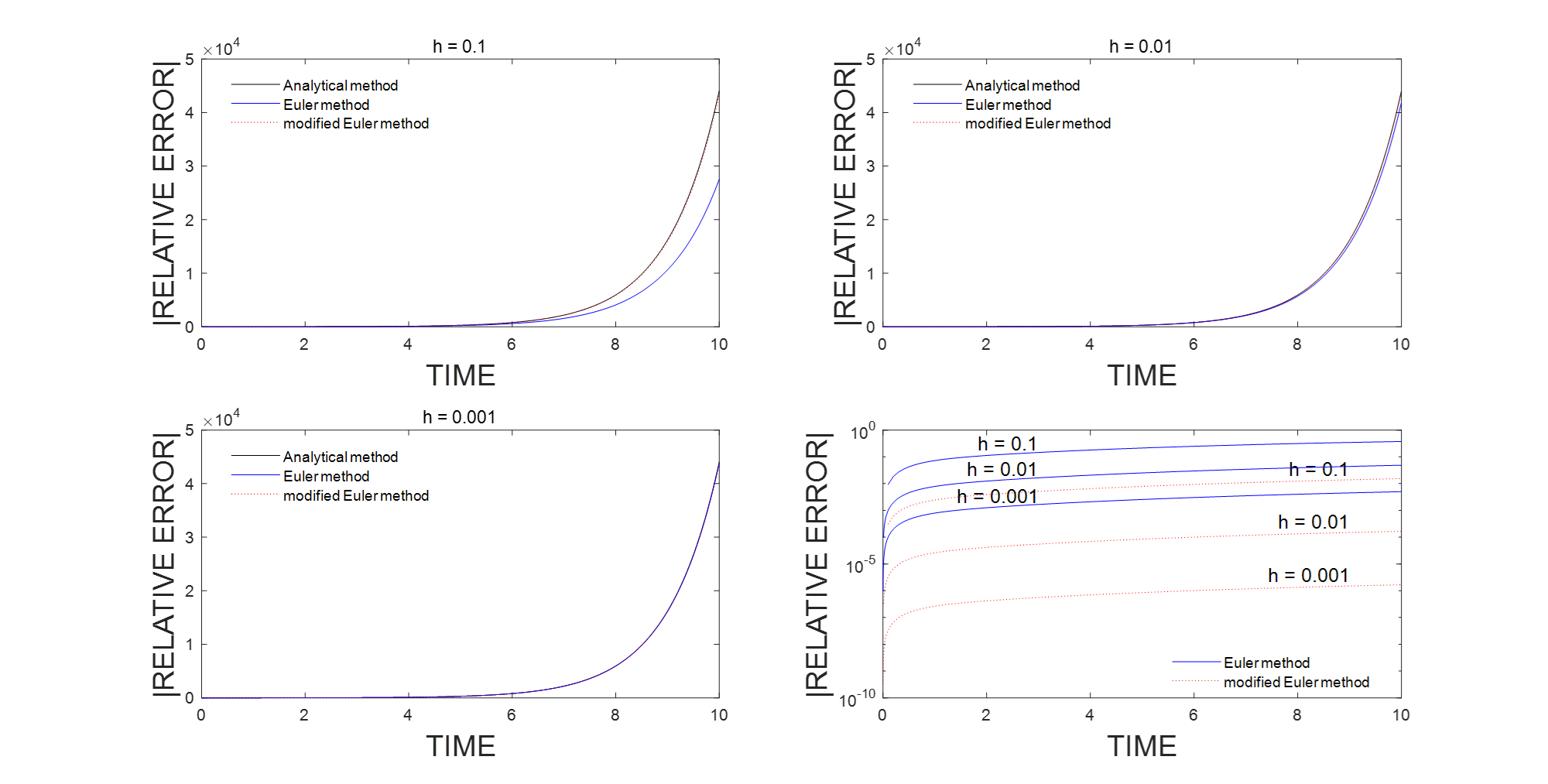
总结和记录一下matlab求解常微分方程常用的数值解法,本文先从欧拉法和改进的欧拉法讲起。
d x d t = f ( x , t ) , x ( t 0 ) = x 0 \frac{d x}{d t}=f(x, t), \quad x\left(t_{0}\right)=x_{0} dtdx=f(x,t),x(t0)=x0
1. 前向欧拉法
前向欧拉法使用了泰勒展开的第一项线性项逼近。
x ( t 0 + h ) = x ( t 0 ) + h x ′ ( t 0 ) + 1 2 x ′ ′ ( ξ ) h 2 , t 0 < ξ < t 0 + h x\left(t_{0}+h\right)=x\left(t_{0}\right)+h x^{\prime}\left(t_{0}\right)+\frac{1}{2} x^{\prime \prime}(\xi) h^{2}, \quad t_{0}<\xi<t_{0}+h x(t0+h)=x(t0)+hx′(t0)+21x′′(ξ)h2,t0<ξ<t0+h
x k + 1 = x k + h x k ′ + O ( h 2 ) = x k + h f ( x k , t k ) + O ( h 2 ) x_{k+1}=x_{k}+h x'_k+O\left(h^{2}\right)=x_{k}+h f\left(x_{k}, t_{k}\right)+O\left(h^{2}\right) xk+1=xk+hxk′+O(h2)=xk+hf(xk,tk)+O(h2)
2. 改进的欧拉法
在原来前向欧拉法的基础上泰勒展开使用了前面两项:
x k + 1 = x k + h x k ′ + 1 2 h 2 x k ′ ′ + O ( h 3 ) x_{k+1}=x_{k}+h x^{\prime}_k+\frac{1}{2} h^{2} x_{k}^{\prime \prime}+O\left(h^{3}\right) xk+1=xk+hxk′+21h2xk′′+O(h3)
这里使用:
x k ′ ′ = x k + 1 ′ − x k ′ h x_{k}^{\prime \prime}=\frac{x_{k+1}^{\prime}-x_{k}^{\prime}}{h} xk′′=hxk+1′−xk′
于是我们有:
x k + 1 = x k + h 2 ( x k ′ + x k + 1 ′ ) + O ( h 3 ) x_{k+1}=x_{k}+\frac{h}{2}\left(x_{k}^{\prime}+x_{k+1}^{\prime}\right)+O\left(h^{3}\right) xk+1=xk+2h(xk′+xk+1′)+O(h3)
也就是:
x k + 1 = x k + h 2 [ f ( x k , t k ) + f ( x k + 1 , t k + 1 ) ] + O ( h 3 ) x_{k+1}=x_{k}+\frac{h}{2}\left[f\left(x_{k}, t_{k}\right)+f\left(x_{k+1}, t_{k+1}\right)\right]+O\left(h^{3}\right) xk+1=xk+2h[f(xk,tk)+f(xk+1,tk+1)]+O(h3)
我们怎么计算 f ( x k + 1 , t k + 1 ) f(x_{k+1},t_{k+1}) f(xk+1,tk+1)呢,因为我们还不知道 x k + 1 x_{k+1} xk+1。
对比前向欧拉法,改进欧拉法的右边不使用 x k + 1 x_{k+1} xk+1(我们还不知道),但是我们可以用前向欧拉法计算的 x k + h f ( x k , t k ) x_{k}+h f\left(x_{k}, t_{k}\right) xk+hf(xk,tk)来代替 x k + 1 x_{k+1} xk+1,于是我们有
x k + 1 = x k + 1 2 ( k 1 + k 2 ) + O ( h 3 ) , 其中: k 1 = h f ( x k , t k ) , k 2 = h f ( x k + h , t k + k 1 ) x_{k+1}=x_{k}+\frac{1}{2}\left(k_{1}+k_{2}\right)+O\left(h^{3}\right), \\\text{其中:}k_{1}=h f\left(x_{k}, t_{k}\right), k_{2}=h f\left(x_{k}+h, t_{k}+k_{1}\right) xk+1=xk+21(k1+k2)+O(h3),其中:k1=hf(xk,tk),k2=hf(xk+h,tk+k1)
对比一下前向欧拉法:
x k + 1 = x k + k 1 + O ( h 2 ) , k 1 = h f ( x k , t k ) x_{k+1}=x_{k}+k_{1}+O\left(h^{2}\right), \quad k_{1}=h f\left(x_{k},t_{k} \right) xk+1=xk+k1+O(h2),k1=hf(xk,tk)
例子
x ′ = x + t , x ( 0 ) = 1 x^{\prime}=x+t, \quad x(0)=1 x′=x+t,x(0)=1
clear% 测试三个不同的步长
test_times = 3;
% 保存时间、差分时间和步长
h_res=ones(1,test_times);
t_res=cell(1,test_times);%时间
tplot_res=cell(1,test_times);%差分的时间,比时间长度少1
% 保存两种数值方法和解析解的计算结果
x_euler_res=cell(1,test_times);
x_modified_res=cell(1,test_times);
x_exact_res=cell(1,test_times);
% 保存误差
diff1_res=cell(1,test_times);
diff2_res=cell(1,test_times);for i = 1:test_times
% 设置步长间隔和步长数
h = 1/10^i; n = 10/h;
% set up initial conditions
t=zeros(n+1,1); t(1) = 0;
x_euler=zeros(n+1,1); x_euler(1) = 1;
x_modified=zeros(n+1,1); x_modified(1) = 1;
x_exact=zeros(n+1,1); x_exact(1) = 1;
% 设置不同的比较误差的图
diff1 = zeros(n,1); diff2 = zeros(n,1); tplot = zeros(n,1);
% define right side of differential equation, Equation 1.7.10
f = inline('xx+tt','tt','xx');
for k = 1:n
t(k+1) = t(k) + h;
% 计算解析解
x_exact(k+1) = 2*exp(t(k+1)) - t(k+1) - 1;
% 使用前向欧拉法计算
k1 = h * f(t(k),x_euler(k));
x_euler(k+1) = x_euler(k) + k1;
tplot(k) = t(k+1);
diff1(k) = x_euler(k+1) - x_exact(k+1);
diff1(k) = abs(diff1(k) / x_exact(k+1));
% 使用改进欧拉法计算
k1 = h * f(t(k),x_modified(k));
k2 = h * f(t(k+1),x_modified(k)+k1);
x_modified(k+1) = x_modified(k) + 0.5*(k1+k2);
diff2(k) = x_modified(k+1) - x_exact(k+1);
diff2(k) = abs(diff2(k) / x_exact(k+1));
end
diff1_res{i}=diff1;
diff2_res{i}=diff2;
tplot_res{i}=tplot;
h_res(i)=h;
x_euler_res{i}=x_euler;
x_modified_res{i}=x_modified;
x_exact_res{i}=x_exact;
t_res{i}=t;
endfigure
for i=1:test_times
subplot(2,2,i)
plot(t_res{i},x_exact_res{i},'k-',t_res{i},x_euler_res{i},'b-',t_res{i},x_modified_res{i},'r:')
xlabel('TIME','Fontsize',18)
ylabel('|RELATIVE ERROR|','Fontsize',18)
legend({'Analytical method','Euler method','modified Euler method'},'Location','best')
legend boxoff;
title(['h = ',num2str(h_res(i))]);
end
subplot(2,2,4)% 计算相对误差
for i=1:test_times
semilogy(tplot_res{i},diff1_res{i},'b-',tplot_res{i},diff2_res{i},'r:')
hold on
num1 = 0.2*10/h_res(i); num2 = 0.8*10/h_res(i);
text(3,diff1_res{i}(num1),['h = ',num2str(h_res(i))],'Fontsize',12,...
'HorizontalAlignment','right',...
'VerticalAlignment','bottom')
text(9,diff2_res{i}(num2),['h = ',num2str(h_res(i))],'Fontsize',12,...
'HorizontalAlignment','right',...
'VerticalAlignment','bottom')
end
xlabel('TIME','Fontsize',18)
ylabel('|RELATIVE ERROR|','Fontsize',18)
legend({'Euler method','modified Euler method'},'Location','best')
legend boxoff;我们对各个不同的步长进行了比较,并比较了它们的误差,发现改进的欧拉法要比前向欧拉法的精度更高。随着步长的变小,误差也在变小。