LeetCode 23 合并 K 个升序链表
来源:力扣(LeetCode)
链接:https://leetcode.cn/problems/merge-k-sorted-lists/description/
博主Github:https://github.com/GDUT-Rp/LeetCode
题目:
给你一个链表数组,每个链表都已经按升序排列。
请你将所有链表合并到一个升序链表中,返回合并后的链表。
示例1:
输入:lists = [[1,4,5],[1,3,4],[2,6]]
输出:[1,1,2,3,4,4,5,6]
解释:链表数组如下:
[1->4->5,1->3->4,2->6
]
将它们合并到一个有序链表中得到。
1->1->2->3->4->4->5->6
示例2:
输入:lists = []
输出:[]
示例3:
输入:lists = [[]]
输出:[]
提示:
- k == lists.length
- 0 <= k <= 1 0 4 10^4 104
- 0 <= lists[i].length <= 500
- − 1 0 4 -10^4 −104 <= lists[i][j] <= 1 0 4 10^4 104
- lists[i] 按 升序 排列
- lists[i].length 的总和不超过 10^4
解题思路:
方法一:顺序合并
用一个变量 ans 来维护以及合并的链表,第 i 次循环把第 i 个链表和 ans 合并,答案保存到 ans 中。
Golang
/*** Definition for singly-linked list.* type ListNode struct {* Val int* Next *ListNode* }*/
func mergeKLists(lists []*ListNode) *ListNode {var ans *ListNodefor i:=0; i<len(lists); i++ {ans = mergeTwoLists(ans, lists[i])}return ans
}func mergeTwoLists(a *ListNode, b *ListNode) *ListNode {if a == nil {return b}if b == nil {return a}var head ListNodetail := &headfor (a != nil && b != nil) {if a.Val < b.Val {tail.Next = aa = a.Next} else {tail.Next = bb = b.Next}tail = tail.Next}if a != nil {tail.Next = a} else {tail.Next = b}return head.Next
}
C++
class Solution {
public:ListNode* mergeTwoLists(ListNode *a, ListNode *b) {if ((!a) || (!b)) return a ? a : b;ListNode head, *tail = &head, *aPtr = a, *bPtr = b;while (aPtr && bPtr) {if (aPtr->val < bPtr->val) {tail->next = aPtr; aPtr = aPtr->next;} else {tail->next = bPtr; bPtr = bPtr->next;}tail = tail->next;}tail->next = (aPtr ? aPtr : bPtr);return head.next;}ListNode* mergeKLists(vector<ListNode*>& lists) {ListNode *ans = nullptr;for (size_t i = 0; i < lists.size(); ++i) {ans = mergeTwoLists(ans, lists[i]);}return ans;}
};
Java
class Solution {public ListNode mergeKLists(ListNode[] lists) {ListNode ans = null;for (int i = 0; i < lists.length; ++i) {ans = mergeTwoLists(ans, lists[i]);}return ans;}public ListNode mergeTwoLists(ListNode a, ListNode b) {if (a == null || b == null) {return a != null ? a : b;}ListNode head = new ListNode(0);ListNode tail = head, aPtr = a, bPtr = b;while (aPtr != null && bPtr != null) {if (aPtr.val < bPtr.val) {tail.next = aPtr;aPtr = aPtr.next;} else {tail.next = bPtr;bPtr = bPtr.next;}tail = tail.next;}tail.next = (aPtr != null ? aPtr : bPtr);return head.next;}
}
复杂度分析
时间复杂度: 假设每个链表的最长长度是 n。在第一次合并后,ans 的长度为 n;第二次合并后,ans 的长度为 2n,第 i 次合并后,ans 的长度为 i×n。第 i 次合并的时间代价是 O(n+(i−1)×n)=O(i×n),那么总的时间代价为 O ( ∑ i = 1 k ( i × n ) ) = O ( ( 1 + k ) ⋅ k 2 × n ) = O ( k 2 n ) O(\sum_{i = 1}^{k} (i \times n)) = O(\frac{(1 + k)\cdot k}{2} \times n) = O(k^2 n) O(∑i=1k(i×n))=O(2(1+k)⋅k×n)=O(k2n),故渐进时间复杂度为 O ( k 2 n ) O(k^2 n) O(k2n)。
空间复杂度 O(1) 。
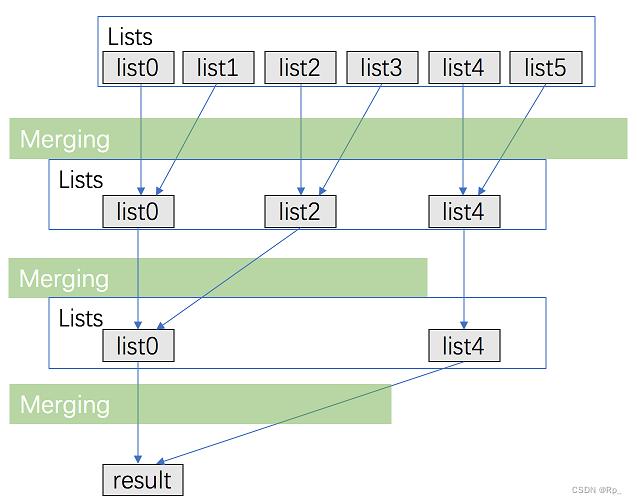
方法二:分治合并
考虑优化方法一,用分治的方法进行合并。
- 将 kkk 个链表配对并将同一对中的链表合并;
- 第一轮合并以后, k 个链表被合并成了 k 2 \frac{k}{2} 2k 个链表,平均长度为 2 n k \frac{2n}{k} k2n,然后是 k 4 \frac{k}{4} 4k 个链表, k 8 \frac{k}{8} 8k 个链表等等;
- 重复这一过程,直到我们得到了最终的有序链表。
Golang
/*** Definition for singly-linked list.* type ListNode struct {* Val int* Next *ListNode* }*/
func mergeKLists(lists []*ListNode) *ListNode {return merge(lists, 0, len(lists) - 1)
}func merge(lists []*ListNode, left, right int) *ListNode {if left == right {return lists[left]}if left > right {return nil}mid := (left + right) >> 1return mergeTwoLists(merge(lists, left, mid), merge(lists, mid+1, right))
}func mergeTwoLists(a *ListNode, b *ListNode) *ListNode {if a == nil {return b}if b == nil {return a}var head ListNodetail := &headfor (a != nil && b != nil) {if a.Val < b.Val {tail.Next = aa = a.Next} else {tail.Next = bb = b.Next}tail = tail.Next}if a != nil {tail.Next = a} else {tail.Next = b}return head.Next
}
C++
class Solution {
public:ListNode* mergeTwoLists(ListNode *a, ListNode *b) {if ((!a) || (!b)) return a ? a : b;ListNode head, *tail = &head, *aPtr = a, *bPtr = b;while (aPtr && bPtr) {if (aPtr->val < bPtr->val) {tail->next = aPtr; aPtr = aPtr->next;} else {tail->next = bPtr; bPtr = bPtr->next;}tail = tail->next;}tail->next = (aPtr ? aPtr : bPtr);return head.next;}ListNode* merge(vector <ListNode*> &lists, int l, int r) {if (l == r) return lists[l];if (l > r) return nullptr;int mid = (l + r) >> 1;return mergeTwoLists(merge(lists, l, mid), merge(lists, mid + 1, r));}ListNode* mergeKLists(vector<ListNode*>& lists) {return merge(lists, 0, lists.size() - 1);}
};
Java
class Solution {public ListNode mergeKLists(ListNode[] lists) {return merge(lists, 0, lists.length - 1);}public ListNode merge(ListNode[] lists, int l, int r) {if (l == r) {return lists[l];}if (l > r) {return null;}int mid = (l + r) >> 1;return mergeTwoLists(merge(lists, l, mid), merge(lists, mid + 1, r));}public ListNode mergeTwoLists(ListNode a, ListNode b) {if (a == null || b == null) {return a != null ? a : b;}ListNode head = new ListNode(0);ListNode tail = head, aPtr = a, bPtr = b;while (aPtr != null && bPtr != null) {if (aPtr.val < bPtr.val) {tail.next = aPtr;aPtr = aPtr.next;} else {tail.next = bPtr;bPtr = bPtr.next;}tail = tail.next;}tail.next = (aPtr != null ? aPtr : bPtr);return head.next;}
}
时间复杂度:考虑递归「向上回升」的过程——第一轮合并 k 2 \frac{k}{2} 2k 组链表,每一组的时间代价是 O ( 2 n ) O(2n) O(2n);第二轮合并 k 4 \frac{k}{4} 4k 组链表,每一组的时间代价是 O ( 4 n ) O(4n) O(4n)…所以总的时间代价是 O ( ∑ i = 1 ∞ k 2 i × 2 i n ) = O ( k n × log k ) O(\sum_{i = 1}^{\infty} \frac{k}{2^i} \times 2^i n) = O(kn \times \log k) O(∑i=1∞2ik×2in)=O(kn×logk),故渐进时间复杂度为 O ( k n × log k ) O(kn \times \log k) O(kn×logk)
空间复杂度:递归会使用到 O ( log k ) O(\log k) O(logk) 空间代价的栈空间。
方法三:使用优先队列/最小堆合并
这个方法和前两种方法的思路有所不同
我们需要维护当前每个链表没有被合并的元素的最前面一个, k k k 个链表就最多有 k k k 个满足这样条件的元素,每次在这些元素里面选取 val 属性最小的元素合并到答案中。
在选取最小元素的时候,我们可以用优先队列/最小堆来优化这个过程。
Golang
/*** Definition for singly-linked list.* type ListNode struct {* Val int* Next *ListNode* }*/import "container/heap"type Status struct {Val intPtr *ListNode
}type PriorityQueue []*Statusfunc (pq PriorityQueue) Len() int {return len(pq)
}func (pq PriorityQueue) Less(i, j int) bool {return pq[i].Val < pq[j].Val
}func (pq PriorityQueue) Swap(i, j int) {pq[i], pq[j] = pq[j], pq[i]
}func (pq *PriorityQueue) Push(x interface{}) {*pq = append(*pq, x.(*Status))
}func (pq *PriorityQueue) Pop() interface{} {old := *pqn := len(old)item := old[n-1]old[n-1] = nil*pq = old[0 : n-1]return item
}func mergeKLists(lists []*ListNode) *ListNode {var q PriorityQueueheap.Init(&q)for _, node := range lists {// 每个链表第一个都放进这个堆if node != nil {heap.Push(&q, &Status{Val: node.Val, Ptr: node})}}var head ListNodetail := &headfor q.Len() > 0 {// 取出最小的f := heap.Pop(&q).(*Status)tail.Next = f.Ptrtail = tail.Next// 只要所取的节点后面还要数据if f.Ptr.Next != nil {// 就放进堆里来heap.Push(&q, &Status{Val: f.Ptr.Next.Val, Ptr: f.Ptr.Next})}}return head.Next
}
Java
class Solution {class Status implements Comparable<Status> {int val;ListNode ptr;Status(int val, ListNode ptr) {this.val = val;this.ptr = ptr;}public int compareTo(Status status2) {return this.val - status2.val;}}PriorityQueue<Status> queue = new PriorityQueue<Status>();public ListNode mergeKLists(ListNode[] lists) {for (ListNode node: lists) {if (node != null) {queue.offer(new Status(node.val, node));}}ListNode head = new ListNode(0);ListNode tail = head;while (!queue.isEmpty()) {Status f = queue.poll();tail.next = f.ptr;tail = tail.next;if (f.ptr.next != null) {queue.offer(new Status(f.ptr.next.val, f.ptr.next));}}return head.next;}
}
C++
class Solution {
public:struct Status {int val;ListNode *ptr;bool operator < (const Status &rhs) const {return val > rhs.val;}};priority_queue <Status> q;ListNode* mergeKLists(vector<ListNode*>& lists) {for (auto node: lists) {if (node) q.push({node->val, node});}ListNode head, *tail = &head;while (!q.empty()) {auto f = q.top(); q.pop();tail->next = f.ptr; tail = tail->next;if (f.ptr->next) q.push({f.ptr->next->val, f.ptr->next});}return head.next;}
};
复杂度分析
时间复杂度:考虑优先队列中的元素不超过 k k k 个,那么插入和删除的时间代价为 ¥O(\log k)$,这里最多有 k n kn kn 个点,对于每个点都被插入删除各一次,故总的时间代价即渐进时间复杂度为 O ( k n × log k ) O(kn \times \log k) O(kn×logk)。
空间复杂度:这里用了优先队列,优先队列中的元素不超过 k k k 个,故渐进空间复杂度为 O ( k ) O(k) O(k)。





![[html]当网站搭建、维护的时候,你会放个什么界面?](https://img-blog.csdnimg.cn/c12ae3d45dd1423aa5c06d1eeaef9bd6.png)