力扣网 110 平衡二叉树

题目描述

给定一个二叉树，判断它是否是高度平衡的二叉树。

本题中，一棵高度平衡二叉树定义为：

一个二叉树每个节点 的左右两个子树的高度差的绝对值不超过 1 。

示例 1：

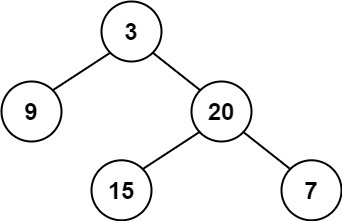
输入：root = [3,9,20,null,null,15,7]
输出：true

示例 2：

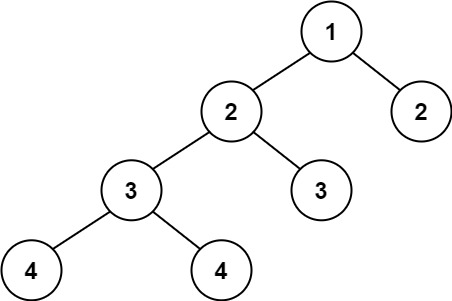
输入：root = [1,2,2,3,3,null,null,4,4]
输出：false

示例 3：

输入：root = []
输出：true

提示：

• 树中的节点数在范围 [0, 5000] 内
• -104 <= Node.val <= 104

思路分析

知识点：递归、二叉树

思路解析：

找出左右子树的高度，如果高度差出现大于一的情况就返回false，从根节点开始，先从左子树找，再去右子树找

这里为了方便判断左右子树高度大小，利用了假设法，先假设左子树高度最高，后面再判断一下，如果不对就换一下。

/*** Definition for a binary tree node.* struct TreeNode {*     int val;*     struct TreeNode *left;*     struct TreeNode *right;* };*/int BinaryTreeHight(struct TreeNode* root)//求二叉树高度
{if (root == NULL){return 0;}return fmax(BinaryTreeHight(root->left), BinaryTreeHight(root->right)) + 1;}
bool isBalanced(struct TreeNode* root) {if(root==NULL){return true;}int left=BinaryTreeHight(root->left);//保存左子树高度int right=BinaryTreeHight(root->right);//保存右子树高度int max=left;//假设法int min=right;if(left<right){min=left;max=right;}if((max-min)>1){return false;}return isBalanced(root->left)&&isBalanced(root->right);}