目录
- 前言
- 我的思路
- 迭代
- 递归
- 结果
- 我的代码
前言
BST(Binary Search Tree)二叉查找树也太简单了吧,今天做的这个有点简单哈哈哈哈。
我的思路
迭代
迭代就是我的主要工作在于更新变量上了,如果我想要找的值比根节点小,我就用根节点的左孩子去替代当前的值。用while循环去控制不为空的条件。
node* search_BST(node* root,char str) {while (root != nullptr) {if (root->info == str) {return root;}else if(root->info <str) {root = root->right;}else if (root->info > str) {root = root->left;}}cout << "此BST中没有这个元素!" << endl;return NULL;
}
递归
递归就是我直接调用自己去找,把我的子树当参数去传递给这个函数。我还是喜欢迭代一点。
node* search_BST_recursion(node* root, char str) {if (root == nullptr) {cout << "此BST中没有这个元素!" << endl;return NULL;}else if (root->info == str) {return root;}else if(root->info < str) {search_BST_recursion(root->right, str);}else {search_BST_recursion(root->left, str);}}
};
我还考虑了一下深拷贝和浅拷贝的问题,一般来说,我们用指针去返回一个对象那基本就是深拷贝了呵呵。
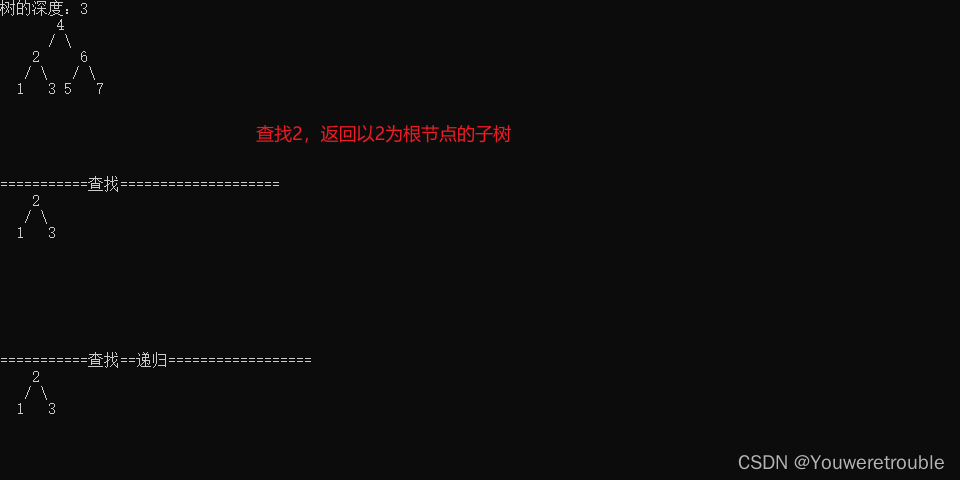
结果

我的代码
#include <iostream>
#include<algorithm>
#include<cmath>
#include <queue>
#include<climits>
using namespace std;struct node {char info;node* left;node* right;node(char data) :info(data), left(nullptr), right(nullptr) {};node() :info(NULL), left(nullptr), right(nullptr) {};
};class binaryTree {
private:node* root;
public:binaryTree() {root = new node(NULL);}//得到树的根结点node* getRoot() {return root;}//得到树的根结点void setRoot(node* newRoot) {root=newRoot;}//以递归的方式构建一棵树void createTree(node*& t,string data,int &i) {char str=data[i];/*cin >> str;*/if (str == '#') {t = NULL;}else {t = new node;//为t开辟空间t->info = str;createTree(t->left,data,++i);createTree(t->right,data,++i);}}//树的深度int depth(node* root) {if (root == nullptr) {return 0;}int left = depth(root->left);int right = depth(root->right);return max(left, right) + 1;}//打印一棵树满二叉树,只能打印满二叉树,节点数目最好不要超过10void print(node*& root) {//存放打印的二叉树char str[10][100] = {};queue<node*> q;int h = depth(root);q.push(root);int index = 0;while (!q.empty()) {int size = q.size();//存放每一层的节点vector<char> list;for (int i = 0; i < size; i++) {node* temp = q.front();q.pop();list.push_back(temp->info);//cout << temp->info;if (temp->left != nullptr) {q.push(temp->left);}if (temp->right != nullptr) {q.push(temp->right);}}bool flag = true;int j = 0;//打印前面部分空白while (j <= 2 * h - 1 - index) {str[index][j] = ' ';j++;}//保持第一行居中if (index == 0) {for (int m = 0; m < h - 2; m++) {str[index][j++] = ' ';}}for (int k = 0; k < list.size(); k++) {//如果是一层最后一个节点if (k == list.size() - 1) {str[index][j++] = list[k];}else {//相邻左右子节点if (k % 2 == 0) {str[index][j++] = list[k];for (int l = 0; l < 3 + 2 * (h - index / 2 - 1); l++) {str[index][j++] = ' ';}}else {str[index][j++] = list[k];str[index][j++] = ' ';}}}index += 2;//cout << endl;}for (int i = 0; i < 10; i++) {if (i % 2 == 1) {for (int j = 0; j < 100; j++) {str[i][j] = ' ';}}}for (int i = 0; i < 10; i++) {if (i % 2 == 0) {for (int j = 0; j < 100; j++) {if (str[i][j] - '0' >= 0 && str[i][j] - '0' <= 9 && i < 2 * h - 2) {str[i + 1][j - 1] = '/';str[i + 1][j + 1] = '\\';}}}}for (int i = 0; i < 10; i++) {for (int j = 0; j < 100; j++) {cout << str[i][j];}cout << endl;}}void DeepFirstSearch(node* root) {if (root == NULL) {return;}else {cout << root->info << ' ';DeepFirstSearch(root->left);DeepFirstSearch(root->right);}}void BreadthFirstSearch(node* root) {queue<node> myTree;if (root != nullptr) {myTree.push(*root);}while (!myTree.empty()) {cout << myTree.front().info << ' ';if (myTree.front().left != nullptr) {myTree.push(*(myTree.front().left));}if (myTree.front().right != nullptr) {myTree.push(*(myTree.front().right));}myTree.pop();}}//用于BFS递归的主函数void BFS_Recursion(node* root, int level, vector<vector<char>>& res) {if (root == nullptr) {return;}if (res.size() < level) {res.push_back(vector<char>());}res[level - 1].push_back(root->info);BFS_Recursion(root->left, level + 1, res);BFS_Recursion(root->right, level + 1, res);}void BreadthFirstSearch_recursion(node* root) {vector<vector<char>> res;BFS_Recursion(root, 1, res);for (int i = 0; i < res.size(); i++) {for (int j = 0; j < res[i].size(); j++) {cout << res[i][j] << " ";}}}//验证是否为二叉搜索树void isBST(node* root) {//先创建一个数组vector<char> midOrderArr;midOrder(root, midOrderArr);//输出看一下我的数组里面存的是不是中序遍历的值for (int i = 0; i < midOrderArr.size(); i++) {cout << midOrderArr[i] << ' ';}cout << endl;for (int i = 0; i < midOrderArr.size() - 1; i++) {if (midOrderArr[i] >= midOrderArr[i + 1]) {cout << "该二叉树 不是一颗二叉搜索树!" << endl;return;}}cout << "该二叉树 是一颗二叉搜索树!" << endl;}//二叉树的中序遍历void midOrder(node* root, vector<char>& Arr) {if (root == nullptr) {return;}midOrder(root->left, Arr);Arr.push_back(root->info);midOrder(root->right, Arr);}bool isBST_Recursion(node* root, long long min, long long max) {if (root == nullptr) {return true;}if (root->info <= min || root->info >= max) {//cout << "该二叉树不是一个二叉搜索树";return false;}return isBST_Recursion(root->left, min, root->info) && isBST_Recursion(root->right, root->info, max);}node* search_BST(node* root,char str) {while (root != nullptr) {if (root->info == str) {return root;}else if(root->info <str) {root = root->right;}else if (root->info > str) {root = root->left;}}cout << "此BST中没有这个元素!" << endl;return NULL;}node* search_BST_recursion(node* root, char str) {if (root == nullptr) {cout << "此BST中没有这个元素!" << endl;return NULL;}else if (root->info == str) {return root;}else if(root->info < str) {search_BST_recursion(root->right, str);}else {search_BST_recursion(root->left, str);}}
};int main() {binaryTree T;node* root = T.getRoot();string data = "421##3##65##7##";string data2 = "1248##9##5##36##7##";char str = '2';int i = 0;T.createTree(root,data,i);cout << "树的深度:" << T.depth(root) << endl;T.print(root);cout << "===========查找====================" << endl;binaryTree resTree;if (T.search_BST(root, str)) {resTree.setRoot(T.search_BST(root, str));node* resTreeRoot = resTree.getRoot();resTree.print(resTreeRoot);}cout << "===========查找==递归==================" << endl;binaryTree resTree2;if (T.search_BST_recursion(root, str)) {resTree2.setRoot(T.search_BST_recursion(root, str));node* resTreeRoot2 = resTree2.getRoot();resTree.print(resTreeRoot2);}return 0;
}