题目: Codeforces Round 942 (Div. 2)
D2有缘再补吧…
A. Contest Proposal
题意
两个升序(不降)的序列a和b,可以在a的任意位置插入任意数(要保持升序),使对任意i,有a[i] <= b[i],问最少插入几次
思路
对于某个位置i插入什么数不用考虑,总有保持升序又满足a[i] <= b[i]的办法的,插入这个操作就相当于把a数组在i之后的部分后移一格
可以从前往后依次插入,这样前边的序列都是满足条件的不用管,后边依次判断要不要插入(如果a[i] > b[i]就要插入)
代码
i是遍历a数组看要不要插入,j是a[i]对应的b数组下标
总之把后移操作换成了移下标,i没动j后移说明a[i]对应的数还在后面,需要后移,答案加一
(我在说什么
#include <iostream>
#include <cstdio>
#include <vector>
#include <cmath>
#include <queue>
#include <cstring>
#include <algorithm>
using namespace std;typedef long long ll;
const int N = 105;int a[N], b[N]; int main()
{int T, n;cin >> T;while (T--){cin >> n;for (int i = 0; i < n; i++)cin >> a[i];for (int i = 0; i < n; i++)cin >> b[i];int ans = 0;int i = 0, j = 0;while (i < n){while (j < n && a[i] > b[j]){j++;ans++;}i++, j++;}cout << ans << endl;}return 0;
}
B. Coin Games
题意
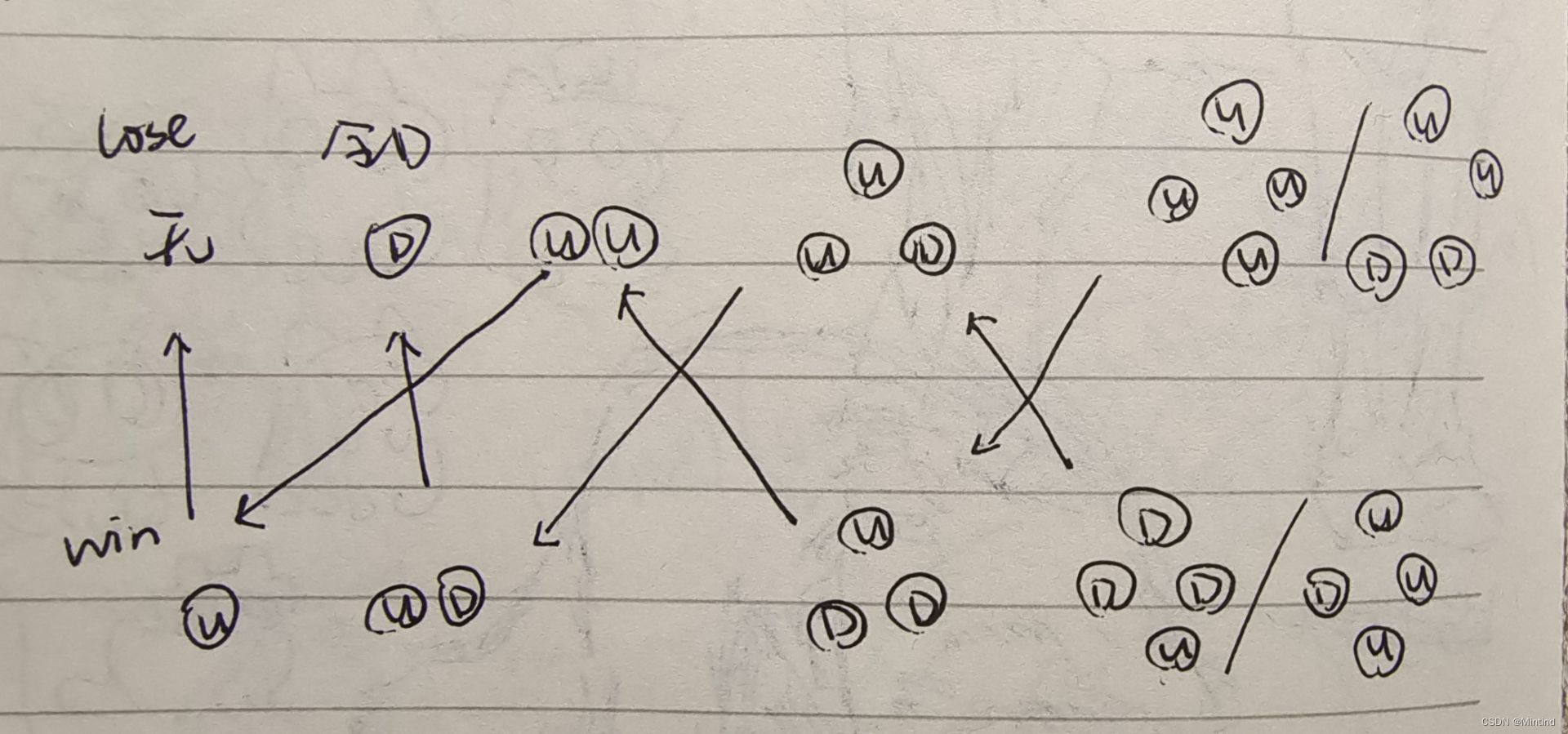
n个硬币,每个硬币有U或者D两个状态(朝上朝下),操作:选一个硬币取走,并把周围两个硬币翻面,玩家A和B依次操作,无法操作判输
思路
列了一些必赢必输的情况,然后发现这不U单数就必赢吗
代码
#include <iostream>
#include <cstdio>
#include <vector>
#include <cmath>
#include <queue>
#include <cstring>
#include <algorithm>
using namespace std;typedef long long ll;
const int N = 105;int a[N], b[N]; int main()
{int T, n;cin >> T;while (T--){cin >> n;string str;cin >> str;int cnt = 0;for (int i = 0; i < str.size(); i++){if (str[i] == 'U') cnt++;}if (cnt & 1) cout << "yes\n";else cout <<"no\n";}return 0;
}
C. Permutation Counting
题意
对i(1…n),你手头有a[i]张写着i的卡,你可以再任意买k张卡(1…n),问最终你手头的卡最多能凑出多少1-n的排列
思路
贪心,首先尽凑出尽可能多套[1-n]
也就是k要先补给数量少的卡 -> 排序,补前边的
枚举要凑出的[1-n]数,判断是否可行 -> 二分答案
假设最多能凑出x个[1-n],先把他们按同样的顺序(这个顺序好像有讲究但是反正不用输出)依次摆开,这样就有x * n - n + 1个排列了
有多的卡(指最后数量>= x + 1)摆在两边,每个数只能摆一次,每摆一个答案加一(总有一个排列顺序↑满足这点),所以答案要加上本来数量就>= x+1的卡 + k补完x个[1-n]后还剩的部分
(剩下的k肯定拿去买数量不够x+1的了,这样答案最大化,而且够不到的卡数一定大于剩下的k,因为小于等于的话都可以再凑一波[1-n]了)
代码
答应我技艺不精就全用long long好吗,在这de半天(给自己一拳
二分,lower_bound,upper_bound我一生之敌
#include <iostream>
#include <cstdio>
#include <vector>
#include <cmath>
#include <queue>
#include <cstring>
#include <algorithm>
#include <vector>
using namespace std;typedef long long ll;
const int N = 2e5 + 5;ll a[N], sum[N];
int n;
ll k;ll cal(ll x)//如果要凑出x个[1-n],需要补多少卡
{int p = lower_bound(a + 1, a + n + 1, x) - a - 1;return x * p - sum[p];
}int main()
{int T;cin >> T;while (T--){cin >> n >> k;for (int i = 1; i <= n; i++)cin >> a[i];sort(a + 1, a + n + 1);//排序!sum[0] = 0;for (int i = 1; i <= n; i++)sum[i] = sum[i - 1] + a[i];//前缀和!好算!ll l = a[1], r = a[1] + k;while (l < r){ll mid = l + r + 1 >> 1;if (cal(mid) <= k) l = mid;else r = mid - 1;}//printf("l %lld\n", l);k = k - cal(l);int p = upper_bound(a + 1, a + n + 1, l) - a - 1;//这里要求第一个本来数量就>= x+1的卡的下标(实际上是p + 1),所以要用upper_boundll res = l * n - n + 1 + k + n - p; //l个[1-n]的 + 剩下的k + 本来就>= x+1的卡数cout << res << endl;}return 0;
}
D1. Reverse Card (Easy Version)
思路
设d = gcd(a, b),a = ka * d, b = kb * d
b * d | a + b
b | ka + kb
kb * d | ka + kb
kb | ka
故有gcd(a, b) = b,设a = k * b
b * b | k * b + b
b | k + 1
设k + 1 = k' * b,则a = (k' * b - 1) * b = k' * b * b - b
对于每个b,有(n + b) / b * b个k’满足a在n范围内
枚举b,算a的个数
(好绕啊…写个博客重推一遍还是没懂思路
代码
#include <iostream>
#include <cstdio>
#include <vector>
#include <cmath>
#include <queue>
#include <cstring>
#include <algorithm>
#include <vector>
using namespace std;typedef long long ll;
const int N = 2e6 + 5;int main()
{ll T, n, m;cin >> T;while (T--){cin >> n >> m;ll ans = n;for (ll i = 2; i <= m; i++){ll t = i * i;ans += (n + i) / t;//if (n/t) cout << i << ' ' << n / t << endl;}cout << ans << endl;}return 0;
}