---

知识总结

今天做了单调栈的三道题
总结了一个模版套路:

1. 寻找下一个更大的数

for(int i = 0; i < len; i++){while(!stack.isEmpty() && nums[i] > nums[stack.peek()]{ans[nums.pop()] = nums[i];}stack.push(i);
}

---

Leetcode 739. 每日温度

题目链接

题目说明

给定一个整数数组 temperatures ，表示每天的温度，返回一个数组 answer ，其中 answer[i] 是指对于第 i 天，下一个更高温度出现在几天后。如果气温在这之后都不会升高，请在该位置用 0 来代替。

代码说明

class Solution {public int[] dailyTemperatures(int[] temperatures) {//单调栈, 存放的数据如果比栈顶元素小, 直接存//如果比栈顶元素大,将小的元素弹出来, 并且记录下结果int[] res = new int[temperatures.length];Deque<Integer> stack = new LinkedList<>();stack.push(0);for(int i = 1; i < temperatures.length; i++){while(!stack.isEmpty() && temperatures[i] > temperatures[stack.peek()]){int index = stack.pop();res[index] = i - index;}stack.push(i);}return res;}
}

---

Leetcode 496. 下一个更大元素 I

题目链接

题目说明

nums1 中数字 x 的 下一个更大元素 是指 x 在 nums2 中对应位置 右侧 的 第一个 比 x 大的元素。

给你两个 没有重复元素 的数组 nums1 和 nums2 ，下标从 0 开始计数，其中nums1 是 nums2 的子集。

对于每个 0 <= i < nums1.length ，找出满足 nums1[i] == nums2[j] 的下标 j ，并且在 nums2 确定 nums2[j] 的 下一个更大元素 。如果不存在下一个更大元素，那么本次查询的答案是 -1 。

返回一个长度为 nums1.length 的数组 ans 作为答案，满足 ans[i] 是如上所述的 下一个更大元素 。

代码说明

这道题和上面的区别在于, 我们需要一个额外的Map 来储存value: index

class Solution {public int[] nextGreaterElement(int[] nums1, int[] nums2) {int len1 = nums1.length, len2 = nums2.length;int[] res = new int[len1];Arrays.fill(res, -1);Map<Integer, Integer> map = new HashMap<>();for(int i = 0; i < len1; i++){map.put(nums1[i], i);}LinkedList<Integer> stack = new LinkedList<>();stack.push(0);for(int i = 0; i < len2; i++){while(!stack.isEmpty() && nums2[i] > nums2[stack.peek()]){int index = stack.pop();if(map.containsKey(nums2[index])){res[map.get(nums2[index])] = nums2[i];}}stack.push(i);}return res;}
}

---

Leetcode 84. 柱状图中最大的矩形

题目链接

题目说明

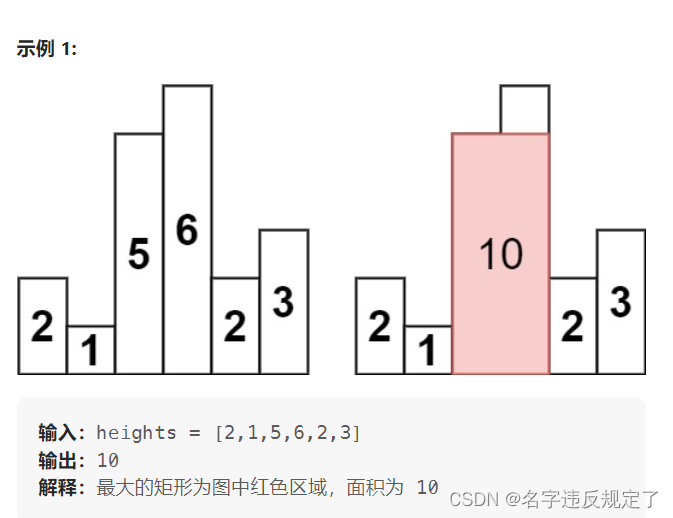
给定 n 个非负整数，用来表示柱状图中各个柱子的高度。每个柱子彼此相邻，且宽度为 1 。

求在该柱状图中，能够勾勒出来的矩形的最大面积。

代码说明

以前做过, 觉得很难, 现在明白了这个套路之后发现很简单.
我们需要两个数组, left, right.
left数组记录以当前高度的左边界, 也就是第一个小于当前高度柱子的index, 默认为-1
同理, right数组记录第一个小于当前数组柱子高度的index. 如果没有则为len

在这个例子中
如果heights = [2, 1, 5, 6, ,2 ,3]
left = [-1, -1, 1, 2, 1, 4]
right = [1, 6, 4, 4, 6, 6]

Area[i] = heights[i] * (right[i] - left[i] - 1)

class Solution {public int largestRectangleArea(int[] heights) {LinkedList<Integer> stack = new LinkedList<>();int len = heights.length;int[] left = new int[len];int[] right = new int[len];// 从左到右, 找到下一个比自己更小的Arrays.fill(right, len);for(int i = 0; i < len; i++){while(!stack.isEmpty() && heights[i] < heights[stack.peek()]){int index = stack.pop();right[index] = i;}stack.push(i);}// System.out.println(Arrays.toString(right));stack.clear();//从右向左遍历, 找到下一个比自己小的Arrays.fill(left, -1);for(int i =  len -1; i>=0;  i--){while(!stack.isEmpty() && heights[i] < heights[stack.peek()]){int index = stack.pop();left[index] = i;}stack.push(i);}// System.out.println(Arrays.toString(left));int res = 0;for(int i = 0; i < len; i++){res = Math.max(heights[i] * (right[i] - left[i] - 1), res);}return res;}
}

---