【数据结构第 6 章 ②】- 用 C 语言实现邻接矩阵-编程知识

一、邻接矩阵表示法

二、AMGraph.h

三、AMGraph.c

四、Test.c

【数据结构第 6 章 ① 】- 图的定义和基本术语-CSDN博客

由于图的结构比较复杂，任意两个顶点之间都可能存在联系，因此无法以数据元素在存储区中的物理位置来表示元素之间的关系，即图没有顺序存储结构，但可以借助二维数组来表示元素之间的关系，即邻接矩阵表示法。另一方面，由于图的任意两个顶点间都可能存在关系，因此，用链式存储表示图是很自然的事，图的链式存储有多种，有邻接表、十字链表和邻接多重表，应根据实际需要的不同，选择不同的存储结构。

一、邻接矩阵表示法

邻接矩阵（Adjacency Matrix）是表示顶点之间相邻关系的矩阵。设 G(V, E) 是具有 n 个顶点的图，则 G 的邻接矩阵是具有如下性质的 n 阶方阵：

例如，图一中的 G1 和 G2 的邻接矩阵如下所示：

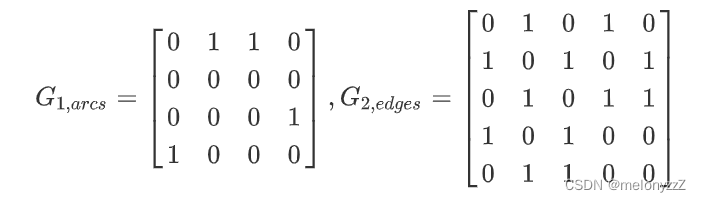
若 G 是网，则邻接矩阵可以定义为：

其中 $w_{i, j}$ 表示边上的权值； $\infty$ 表示计算机允许的，大于所有边上权值的数。例如，下图所示为一个有向网和它的邻接矩阵。

二、AMGraph.h

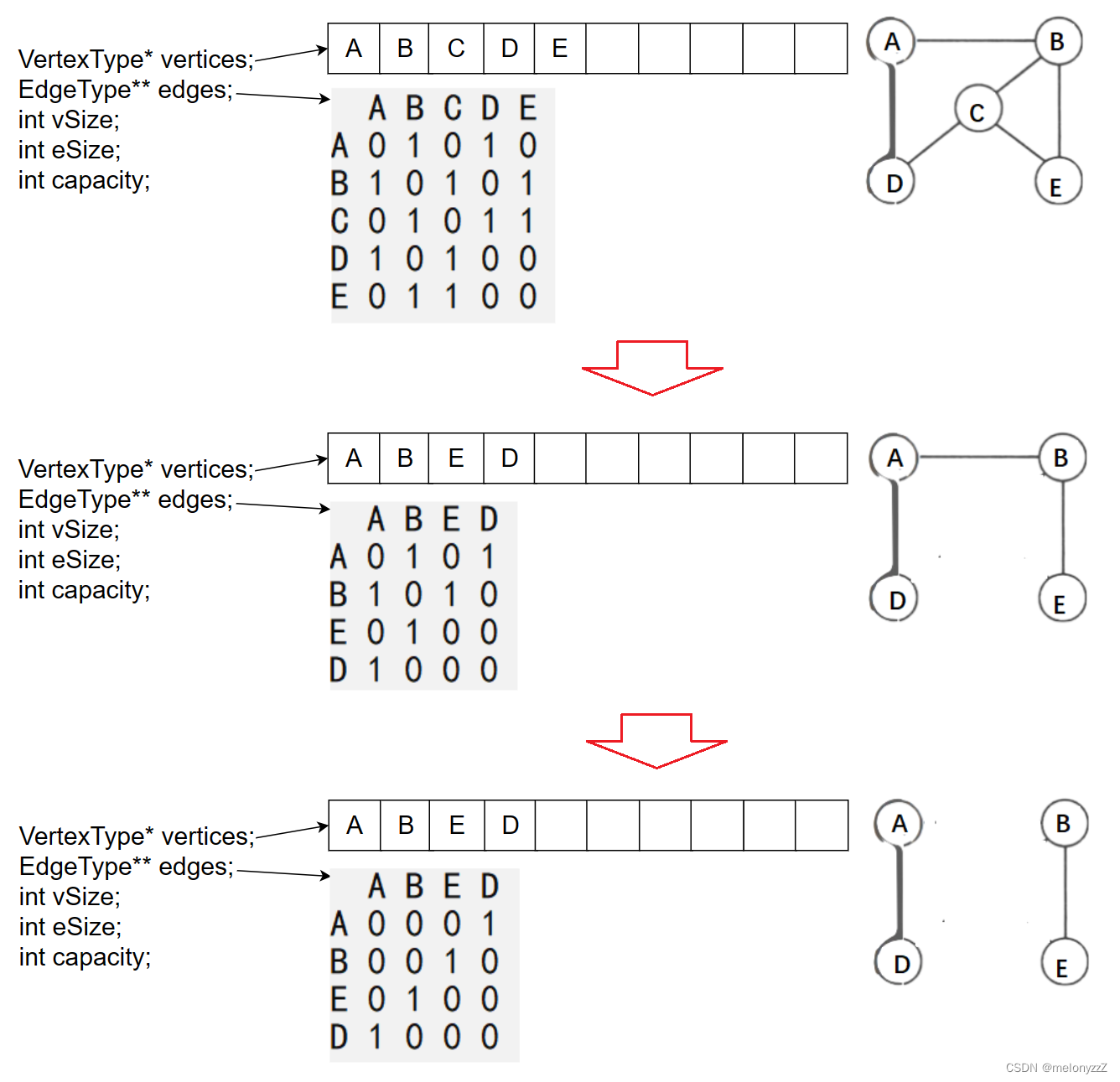
用邻接矩阵表示法表示图，除了一个用于存储邻接矩阵的二维数组外，还需要用一个一维数组来存储顶点信息。

注意：下面是以无向图为例的。

#pragma once#define DEFAULT_CAPACITY 10typedef char VertexType;  // 假定顶点的数据类型为 char
typedef int EdgeType;  // 假定边的权值的数据类型为 inttypedef struct AMGraph
{VertexType* vertices;  // 顶点表（vertices 是 vertex 的复数）EdgeType** edges;  // 邻接矩阵int vSize;  // 当前图中的顶点数int eSize;  // 当前图中的边数int capacity;  // 容量
}AMGraph;// 基本操作
void AMGraphInit(AMGraph* pg);  // 初始化void ShowAdjMatrix(AMGraph* pg);  // 显示邻接矩阵int GetVetexPos(AMGraph* pg, VertexType v);  // 获取顶点的位置void InsertVertex(AMGraph* pg, VertexType v);  // 插入顶点
void InsertEdge(AMGraph* pg, VertexType v1, VertexType v2);  // 插入边void EraseVertex(AMGraph* pg, VertexType v);  // 删除顶点
void EraseEdge(AMGraph* pg, VertexType v1, VertexType v2);  // 删除边int GetFirstAdjVexPos(AMGraph* pg, VertexType v);  // 获取 v 的第一个邻接顶点的位置
int GetNextAdjVexPos(AMGraph* pg, VertexType v, VertexType w);
// 获取 v 的（相对于 w 的）下一个邻接顶点的位置void AMGraphDestroy(AMGraph* pg);  // 销毁

三、AMGraph.c

初始化：

void AMGraphInit(AMGraph* pg)
{assert(pg);pg->vSize = pg->eSize = 0;pg->capacity = DEFAULT_CAPACITY;pg->vertices = (VertexType*)malloc(sizeof(VertexType) * pg->capacity);assert(pg->vertices);pg->edges = (EdgeType**)malloc(sizeof(EdgeType*) * pg->capacity);assert(pg->edges);for (int i = 0; i < pg->capacity; ++i){pg->edges[i] = (EdgeType*)malloc(sizeof(EdgeType) * pg->capacity);assert(pg->edges[i]);for (int j = 0; j < pg->capacity; ++j){pg->edges[i][j] = 0;}}
}

获取顶点的位置：

int GetVetexPos(AMGraph* pg, VertexType v)
{assert(pg);for (int i = 0; i < pg->vSize; ++i){if (pg->vertices[i] == v)return i;}return -1;
}

显示邻接矩阵：

void ShowAdjMatrix(AMGraph* pg)
{assert(pg);printf("  ");  // 输出两个空格for (int i = 0; i < pg->vSize; ++i){printf("%c ", pg->vertices[i]);}printf("\n");for (int i = 0; i < pg->vSize; ++i){printf("%c ", pg->vertices[i]);for (int j = 0; j < pg->vSize; ++j){printf("%d ", pg->edges[i][j]);}printf("\n");}
}

插入顶点：

void InsertVertex(AMGraph* pg, VertexType v)
{assert(pg);// 考虑是否需要扩容if (pg->vSize == pg->capacity){VertexType* tmp1 = (VertexType*)realloc(pg->vertices, sizeof(VertexType) * 2 * pg->capacity);assert(tmp1);pg->vertices = tmp1;EdgeType** tmp2 = (EdgeType**)realloc(pg->edges, sizeof(EdgeType*) * 2 * pg->capacity);assert(tmp2);pg->edges = tmp2;for (int i = 0; i < pg->capacity; ++i){EdgeType* tmp3 = (EdgeType*)realloc(pg->edges[i], sizeof(EdgeType) * 2 * pg->capacity);assert(tmp3);pg->edges[i] = tmp3;for (int j = pg->capacity; j < 2 * pg->capacity; ++j){pg->edges[i][j] = 0;}}for (int i = pg->capacity; i < 2 * pg->capacity; ++i){pg->edges[i] = (EdgeType*)malloc(sizeof(EdgeType) * 2 * pg->capacity);assert(pg->edges[i]);for (int j = 0; j < 2 * pg->capacity; ++j){pg->edges[i][j] = 0;}}pg->capacity *= 2;}// 插入顶点pg->vertices[pg->vSize++] = v;
}

插入边：

void InsertEdge(AMGraph* pg, VertexType v1, VertexType v2)
{assert(pg);int pos1 = GetVetexPos(pg, v1);int pos2 = GetVetexPos(pg, v2);if (pos1 == -1 || pos2 == -1)return;if (pg->edges[pos1][pos2] != 0)return;pg->edges[pos1][pos2] = pg->edges[pos2][pos1] = 1;++pg->eSize;
}

删除顶点：

void EraseVertex(AMGraph* pg, VertexType v)
{assert(pg);int pos = GetVetexPos(pg, v);if (pos == -1)return;// cnt 为和 v 相关联的边的数目int cnt = 0;for (int j = 0; j < pg->vSize; ++j){if (pg->edges[pos][j] != 0)++cnt;}pg->vertices[pos] = pg->vertices[pg->vSize - 1];for (int j = 0; j < pg->vSize; ++j){pg->edges[pos][j] = pg->edges[pg->vSize - 1][j];}for (int i = 0; i < pg->vSize; ++i){pg->edges[i][pos] = pg->edges[i][pg->vSize - 1];}--pg->vSize;pg->eSize -= cnt;
}

删除边：

void EraseEdge(AMGraph* pg, VertexType v1, VertexType v2)
{assert(pg);int pos1 = GetVetexPos(pg, v1);int pos2 = GetVetexPos(pg, v2);if (pos1 == -1 || pos2 == -1)return;if (pg->edges[pos1][pos2] == 0)return;pg->edges[pos1][pos2] = pg->edges[pos2][pos1] = 0;--pg->eSize;
}

获取 v 的第一个邻接顶点：

int GetFirstAdjVexPos(AMGraph* pg, VertexType v)
{assert(pg);int pos = GetVetexPos(pg, v);if (pos == -1)return -1;for (int j = 0; j < pg->vSize; ++j){if (pg->edges[pos][j] != 0)return j;}return -1;
}

获取 v 的（相对于 w 的）下一个邻接顶点：

int GetNextAdjVexPos(AMGraph* pg, VertexType v, VertexType w)
{assert(pg);int pos1 = GetVetexPos(pg, v);int pos2 = GetVetexPos(pg, w);if (pos1 == -1 || pos2 == -1)return -1;for (int j = pos2 + 1; j < pg->vSize; ++j){if (pg->edges[pos1][j] != 0)return j;}return -1;
}

销毁：

void AMGraphDestroy(AMGraph* pg)
{assert(pg);free(pg->vertices);pg->vertices = NULL;for (int i = 0; i < pg->capacity; ++i){free(pg->edges[i]);pg->edges[i] = NULL;}free(pg->edges);pg->edges = NULL;pg->vSize = pg->eSize = pg->capacity = 0;
}

四、Test.c

#include "AMGraph.h"
#include <stdio.h>int main()
{AMGraph g;AMGraphInit(&g); InsertVertex(&g, 'A');InsertVertex(&g, 'B');InsertVertex(&g, 'C');InsertVertex(&g, 'D');InsertVertex(&g, 'E');InsertEdge(&g, 'A', 'B');InsertEdge(&g, 'A', 'D');InsertEdge(&g, 'B', 'C');InsertEdge(&g, 'B', 'E');InsertEdge(&g, 'C', 'D');InsertEdge(&g, 'C', 'E');ShowAdjMatrix(&g);printf("\n");EraseVertex(&g, 'C');ShowAdjMatrix(&g);printf("\n");EraseEdge(&g, 'A', 'B');ShowAdjMatrix(&g);printf("\n");printf("%d\n", GetFirstAdjVexPos(&g, 'A'));  // 3printf("%d\n", GetNextAdjVexPos(&g, 'A', 'D'));  // -1AMGraphDestroy(&g);return 0;
}