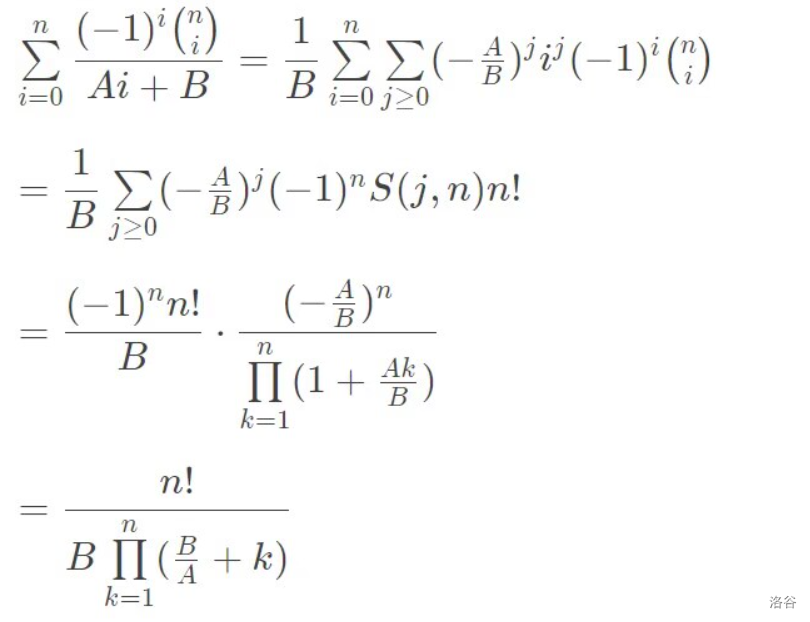题目1513. 找树左下角的值
给定一个二叉树的 根节点 root,请找出该二叉树的 最底层 最左边 节点的值。
假设二叉树中至少有一个节点。
示例 1:
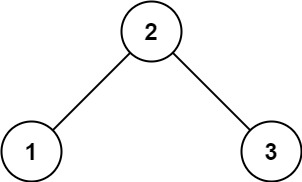
输入: root = [2,1,3]
输出: 1
示例 2:
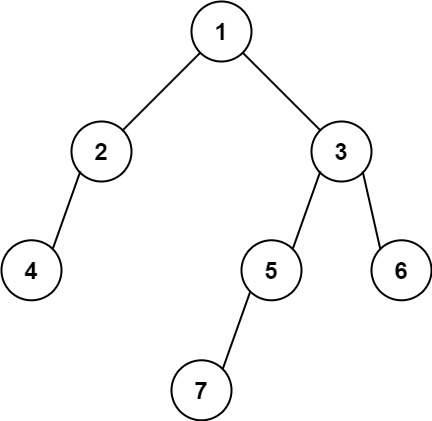
输入: [1,2,3,4,null,5,6,null,null,7]
输出: 7
提示:
- 二叉树的节点个数的范围是
[1,104] -231 <= Node.val <= 231 - 1
思路
迭代法
用辅助queue进行层序遍历就行了,直到最后一层遍历完,取出最左边的节点。
代码
class Solution {
public:int findBottomLeftValue(TreeNode* root) {if(!root->left && !root->right){return root->val;}queue<TreeNode*> nodeQueue;TreeNode* result;nodeQueue.push(root);while(!nodeQueue.empty()){int num = nodeQueue.size();if(num > 0){result = nodeQueue.front();}for(int i = 0; i < num; i++){TreeNode* curNode = nodeQueue.front();nodeQueue.pop();if(curNode->left){nodeQueue.push(curNode->left);}if(curNode->right){nodeQueue.push(curNode->right);}}}return result->val;}
};
递归法
用辅助变量maxdepth和depth来判断叶子节点是否是左下角的节点,其他的部分就先序遍历做就行了。
代码
class Solution {
public:int result;int maxDepth;void getResult(TreeNode* root, int depth){//碰到叶子节点判断是否为最深的左叶子if(!root->left && !root->right){if(depth > maxDepth){maxDepth = depth;result = root->val;}return;}if(root->left){getResult(root->left, depth + 1);}if(root->right)getResult(root->right, depth + 1);}int findBottomLeftValue(TreeNode* root) {maxDepth = INT_MIN;getResult(root, 0);return result;}
};
题目2 112. 路径总和
给你二叉树的根节点 root 和一个表示目标和的整数 targetSum 。判断该树中是否存在 根节点到叶子节点 的路径,这条路径上所有节点值相加等于目标和 targetSum 。如果存在,返回 true ;否则,返回 false 。
叶子节点 是指没有子节点的节点。
示例 1:
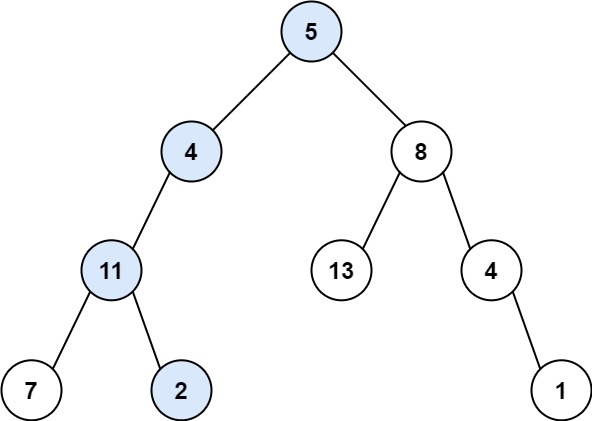
输入:root = [5,4,8,11,null,13,4,7,2,null,null,null,1], targetSum = 22
输出:true
解释:等于目标和的根节点到叶节点路径如上图所示。
示例 2:
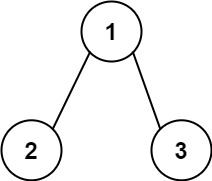
输入:root = [1,2,3], targetSum = 5
输出:false
解释:树中存在两条根节点到叶子节点的路径:
(1 --> 2): 和为 3
(1 --> 3): 和为 4
不存在 sum = 5 的根节点到叶子节点的路径。
示例 3:
输入:root = [], targetSum = 0
输出:false
解释:由于树是空的,所以不存在根节点到叶子节点的路径。
提示:
- 树中节点的数目在范围
[0, 5000]内 -1000 <= Node.val <= 1000-1000 <= targetSum <= 1000
思路
递归法
用先序遍历,注意节点是否为叶子节点就行了。
代码
class Solution {
public:bool hasPathSum(TreeNode* root, int targetSum) {if(root == nullptr){return false;}int curVal = targetSum - root->val;if(curVal == 0 && !root->left && !root->right){return true;}return hasPathSum(root->left, curVal) || hasPathSum(root->right, curVal); }
};
迭代法
我使用一个辅助栈stack<pair<TreeNode*, int>>对象来保存每个节点及剩余的路径,剩下的用什么遍历都可以找出目标叶子节点。
代码
class Solution {
public:bool hasPathSum(TreeNode* root, int targetSum) {if(root == nullptr){return false;}stack<pair<TreeNode*, int>> nodeStack;nodeStack.push(make_pair(root, targetSum));while(!nodeStack.empty()){auto iter = nodeStack.top();nodeStack.pop();if(!iter.first->left && !iter.first->right && iter.first->val == iter.second){return true;}if(iter.first->left){nodeStack.push(make_pair(iter.first->left, iter.second - iter.first->val));}if(iter.first->right){nodeStack.push(make_pair(iter.first->right, iter.second - iter.first->val));}}return false;}
};
题目3 106. 从中序与后序遍历序列构造二叉树
给定两个整数数组 inorder 和 postorder ,其中 inorder 是二叉树的中序遍历, postorder 是同一棵树的后序遍历,请你构造并返回这颗 二叉树 。
示例 1:
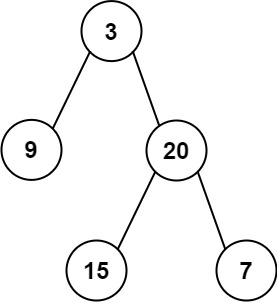
输入:inorder = [9,3,15,20,7], postorder = [9,15,7,20,3]
输出:[3,9,20,null,null,15,7]
示例 2:
输入:inorder = [-1], postorder = [-1]
输出:[-1]
迭代法
用的迭代法,思路和代码随想里的递归法类似。
代码
class Solution {
public:TreeNode* buildTree(vector<int>& inorder, vector<int>& postorder) {if (inorder.empty() || postorder.empty()) return nullptr;// 根节点TreeNode* root = new TreeNode(postorder.back());postorder.pop_back(); // 删除最后一个元素,因为这是根节点// 找到根节点在 inorder 中的索引int rootIndex = 0;while (inorder[rootIndex] != root->val) {rootIndex++;}// 栈保存:parent节点,inorder左边界,inorder右边界,postorder左边界,postorder右边界,是否为左子树stack<tuple<TreeNode*, int, int, int, int, bool>> nodeStack;// 处理右子树if (rootIndex + 1 <= inorder.size() - 1) {nodeStack.push(make_tuple(root, rootIndex + 1, inorder.size() - 1, rootIndex, postorder.size() - 1, false));}// 处理左子树if (0 <= rootIndex - 1) {nodeStack.push(make_tuple(root, 0, rootIndex - 1, 0, rootIndex - 1, true));}// 遍历栈while (!nodeStack.empty()) {auto [parent, inStart, inEnd, postStart, postEnd, isLeft] = nodeStack.top();nodeStack.pop();// 新的根节点是后序遍历 postorder[postEnd] 的值TreeNode* curNode = new TreeNode(postorder[postEnd]);if (isLeft) {parent->left = curNode;} else {parent->right = curNode;}// 在 inorder 中找到当前节点的索引int inIndex = inStart;while (inorder[inIndex] != curNode->val) {inIndex++;}// 处理右子树if (inIndex + 1 <= inEnd) {nodeStack.push(make_tuple(curNode, inIndex + 1, inEnd, postEnd - (inEnd - inIndex), postEnd - 1, false));}// 处理左子树if (inStart <= inIndex - 1) {nodeStack.push(make_tuple(curNode, inStart, inIndex - 1, postStart, postStart + (inIndex - inStart) - 1, true));}}return root;}
};