目录
- 1.字母大小写全排列
- 1.题目链接
- 2.算法原理详解
- 3.代码实现
- 2.优美的排列
- 1.题目链接
- 2.算法原理详解
- 3.代码实现
- 3.N 皇后
- 1.题目链接
- 2.算法原理详解
- 3.代码实现
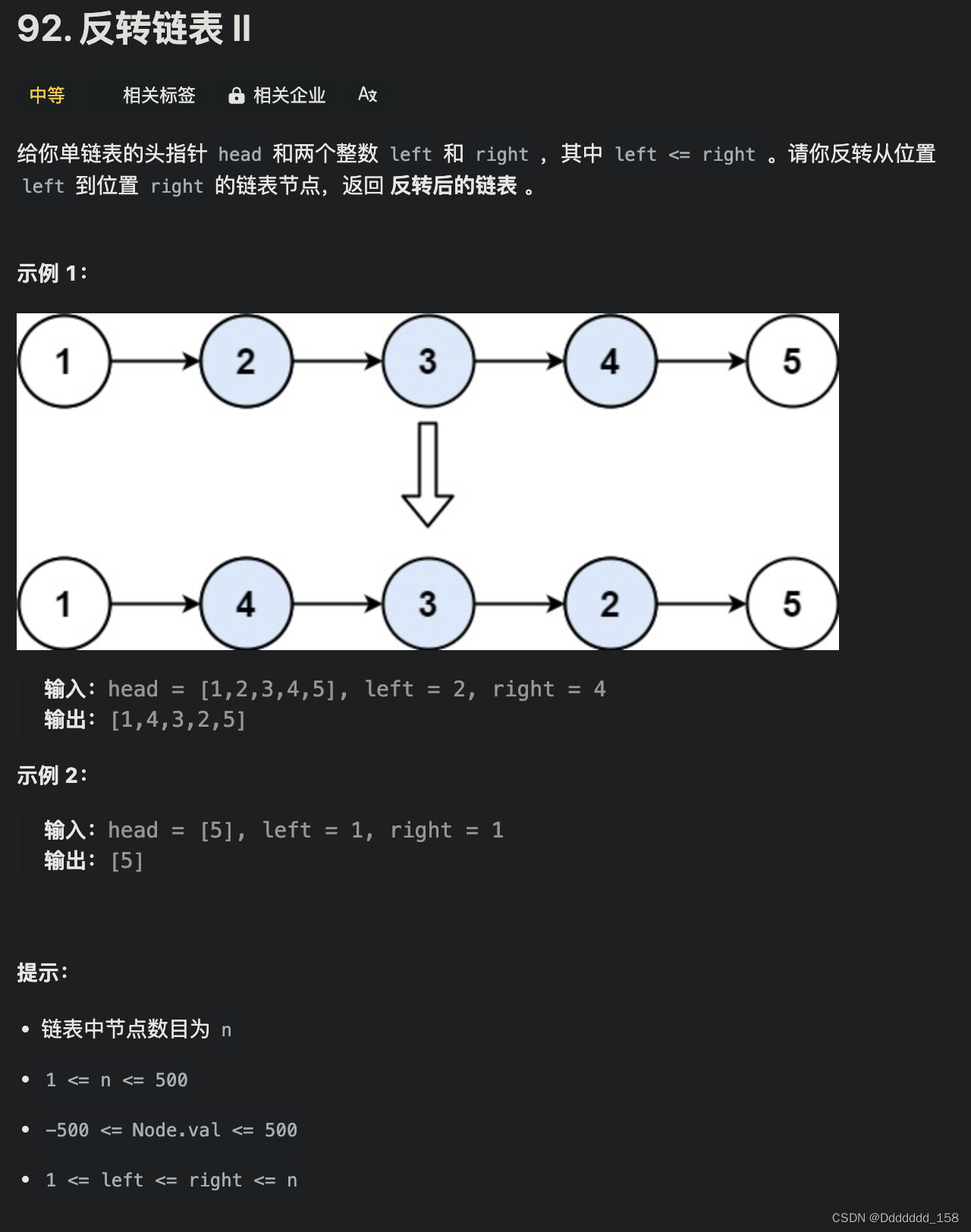
1.字母大小写全排列
1.题目链接
- 字母大小写全排列
2.算法原理详解
- 本题逻辑与子集大致相同
-
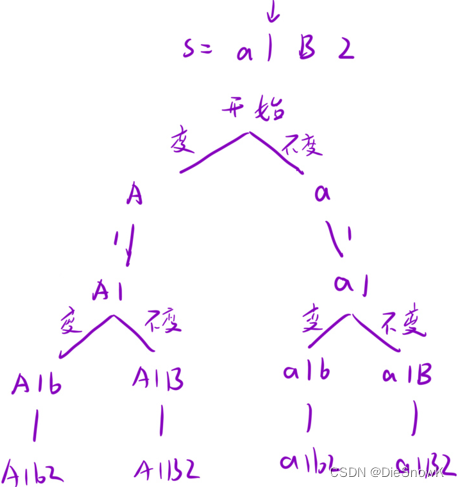
- 思路一:每次盯着一个字符,变或是不变
- 全局变量:
string pathvector<string> ret
DFS()设计- 函数头:
void DFS(string, pos)pos:下一层递归要选的元素
- 函数体:
- 字母可能变/不变,数字一定不需要变
- 递归出口:
pos == nums.size()
- 函数头:
- 回溯:变完函数返回时需要回溯
- 全局变量:
- 思路一:每次盯着一个字符,变或是不变
3.代码实现
class Solution
{string path;vector<string> ret;
public:vector<string> letterCasePermutation(string s) {DFS(s, 0);return ret;}void DFS(string& s, int pos){if(pos == s.size()){ret.push_back(path);return;}char ch = s[pos];// 不改变path += ch;DFS(s, pos + 1);path.pop_back(); // 回溯,恢复现场// 改变if(ch < '0' || ch > '9'){ch = Change(ch);path += ch;DFS(s, pos + 1);path.pop_back(); // 回溯,恢复现场}}char Change(char ch){if(ch >= 'a' && ch <= 'z'){ch -= 32;}else{ch += 32;}return ch;}
};
2.优美的排列
1.题目链接
- 优美的排列
2.算法原理详解
- 思路:对每个位置挨个尝试填入数字
- 全局变量:
int retvector<bool> check-> 剪枝
DFS()设计:void DFS(pos, n)- 剪枝:
- 之前用过的数字不再使用
- 不符合情况的不填入
- 回溯:每层递归返回时回溯
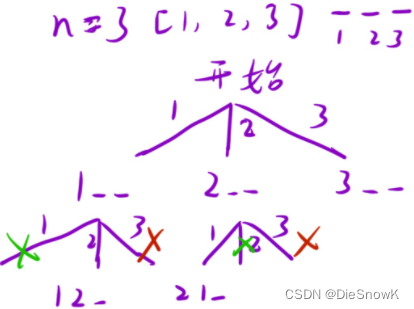
- 全局变量:
3.代码实现
class Solution
{int ret = 0;vector<bool> check;
public:int countArrangement(int n) {check.resize(n + 1, false);DFS(1, n);return ret;}void DFS(int pos, int n){if(pos == n + 1){ret++;return;}for(int i = 1; i <= n; i++){if(!check[i] && (i % pos == 0 || pos % i == 0)){check[i] = true;DFS(pos + 1, n);check[i] = false; // 回溯,恢复现场}}}
};
3.N 皇后
1.题目链接
- N 皇后
2.算法原理详解
-
本题可以学习二维数组判断行列、主副对角线是否放有数据
-
思路:在每一行找合适的列放置皇后,即每次枚举都是枚举一行
-DFS()设计:void DFS(row)- 决策树
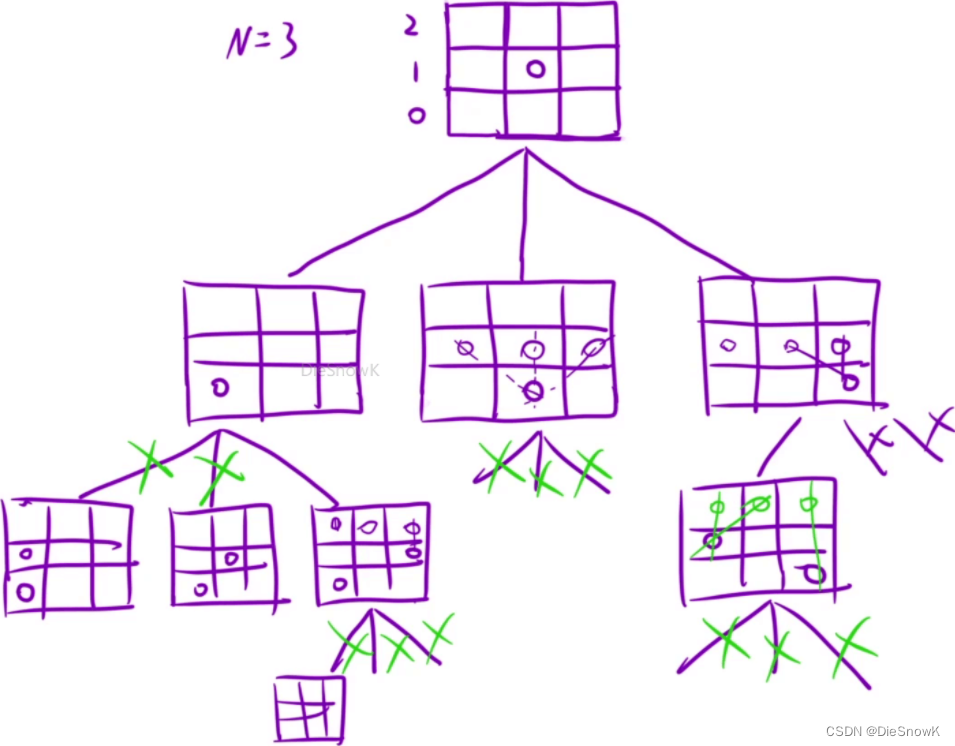
- 决策树
-
如何剪枝?-> 当前这个位置,能否放上皇后?
- 无脑四个循环判断行列、主副对角线 -> ×
- 类似哈希表的策略,需要一定数学理解
- 行不需要剪枝,收递归限制
bool checkCol[n]-> 判断列- 对应下标表示每列是否放置过皇后
bool checkDig1[2 * n]-> 主对角线y = x + b->y - x = b->b可以唯一标识一个对角线y - x + n = b + n-> 两边加上一个固有偏移量防止下标出现负数
bool checkDig2[2 * n]-> 副对角线y = -x + b->y + x = b->b可以唯一标识一个对角线- 副对角线不需要固定偏移量,因为副对角线的纵截距都大于0

3.代码实现
class Solution
{int _n = 0;vector<bool> checkCol;vector<bool> checkDig1;vector<bool> checkDig2;vector<vector<string>> ret;vector<string> path;
public:vector<vector<string>> solveNQueens(int n) {_n = n;checkCol.resize(n, false);checkDig1.resize(2 * n, false);checkDig2.resize(2 * n, false);path.resize(n, string(n, '.'));DFS(0);return ret;}void DFS(int row){// 递归出口if(row == _n){ret.push_back(path);return;}// 对于每一行,枚举每一列for(int i = 0; i < _n; i++){// 剪枝if(!checkCol[i] && !checkDig1[row - i + _n] && !checkDig2[row + i]){checkCol[i] = checkDig1[row - i + _n] = checkDig2[row + i] = true;path[row][i] = 'Q';DFS(row + 1);checkCol[i] = checkDig1[row - i + _n] = checkDig2[row + i] = false; // 回溯path[row][i] = '.';}}}
};